E.J. Green, Rutgers University
[Jump to John Hummel’s commentary]
[Jump to Jake Quilty-Dunn’s commentary]
1. Introduction
Imagine that you are meeting a friend for coffee, and you see her walking toward your table. As she walks, her arms and legs turn about their joints. Moreover, her forearms turn slightly about her elbows, and her tibias move about her knees. I suggest that, despite these changes, her overall structure seems to remain stable. Call this phenomenon structure constancy. Structure constancy is ubiquitous in our visual experiences of objects. In this paper I’ll offer an account of structure constancy, and then I’ll argue that the phenomenon has important consequences for viable theories of the subpersonal underpinnings of visual spatial phenomenology.
I’ll begin in section 2 with a general discussion of perceptual constancy, and then I’ll identify an important respect in which structure constancy differs from the more familiar geometrical constancies. In section 3, I’ll offer a characterization of compositional structure, and argue that structure constancy involves experientially representing an object as retaining compositional structure across certain geometrical changes. In section 4, I’ll argue that the phenomenology of structure constancy cannot be underpinned by a representational format that fails to make part structure explicit, and that this has implications for identifying the locus of visual shape phenomenology within visual system processing. In section 5, I’ll argue that structure constancy raises a problem for views on which the visual representation that underlies our experience of spatial/geometrical properties is wholly viewer-centered. I suggest that our visual experience of geometrical properties plausibly reflects the simultaneous deployment of multiple reference frames for specifying location.
2. Perceptual Constancy
2.1. What is perceptual constancy?
Most theorists agree that perceptual constancy involves a type of stability in one’s perceptual response across certain changes (cf. Cohen forthcoming). Thus, Tyler Burge (2010) writes: “Perceptual constancies are capacities systematically to represent a particular or an attribute as the same despite significant variations in registration of proximal stimulation” (408). Similarly, Stephen Palmer characterizes (visual) perceptual constancy as “the ability to perceive the properties of environmental objects, which are largely constant over different viewing conditions, rather than the properties of their projected retinal images, which vary greatly with viewing conditions” (Palmer 1999: 125).[1]
Under these characterizations, to display perceptual constancy with respect to a property P, one must at minimum perceptually represent P across changes in the way one’s sensory organs are stimulated. In the case of vision, this would be to perceptually represent P across changes in the stimulation of retinal cells.[2]
While Burge’s definition provides a useful starting point, it has a significant drawback. Burge does not say what is involved in representing a particular or attribute “as the same” across variations in proximal stimulation. On one reading, this would require that a subject (or a perceptual system) represent that something perceived under one condition of proximal stimulation is the same—or, at least, the same in respect of a particular attribute, such as color—as something perceived under a different condition of proximal stimulation. On another reading, it would require only that one perceptually attribute the same property P to individuals encountered under different conditions of proximal stimulation.
The first notion is more demanding. To represent that things perceived under different conditions are the same in respect of a particular property, one must be able to perceptually represent comparisons or relations between those things. This might involve either representing that some property P is shared by things perceived in different conditions, or retained by a single thing perceived in different conditions. There is no such requirement in order to simply represent the same property P under two different conditions. We can call the first notion the strong type of constancy, and the latter the weak type. I’ll suggest below that structure constancy is generally of the strong type.
2.2. Geometrical constancies
To set up the rest of the paper, I want to briefly apply this account to geometrical constancies in particular. I’ll understand an object’s “geometrical properties” to include its size, shape, and location. Moreover, I’ll henceforth focus on the strong type of perceptual constancy, where one not only recovers a property under two different conditions, but also represents that the property is shared or retained across changes in proximal stimulation.
To delineate the nature of geometrical constancy under this characterization, we need to know what features—or “cues”—within proximal stimulation are relevant to recovering distal geometrical properties. Research indicates that in the case of shape and size perception, there are a number of such cues—e.g., 2-D retinal shape, context, shading, texture, and motion, among others (Palmer 1999: ch. 5). For the sake of simplicity, however, let’s just focus on 2-D retinal shape. Accordingly, our paradigm case of geometrical constancy in what follows will be one in which a subject perceptually represents an object as retaining a geometrical property (distal shape or size) across changes in the shape or size of its retinal projection.
Changes in the shape or size of an object’s retinal projection result from transformations of the object within a retinocentric frame of reference (a frame of reference built around an origin and intrinsic axes of the retina). For instance, if the object undergoes a rotation transformation where it is slanted in depth relative to the line of sight, this issues in a change in the shape of its projection on the retina. A circular object presents a circular image when seen straight on, but an elliptical image when seen at a slant.
Geometers classify transformations as rigid or non-rigid. Rigid transformations are ones that don’t involve any changes to an object’s intrinsic metric properties. By the “metric properties” of an object, I have in mind, roughly, those properties of the object that depend essentially on its constituent edge lengths, angles, and curvature. For instance, a metric property of a square surface is the property of having four angles of 90°. Rigid transformations include translation (simple change of position), rotation, and reflection (change in “handedness”). Such transformations do not alter the distances or angles between points of the transformed object.
Non-rigid transformations, on the other hand, do involve changes to an object’s intrinsic metric properties. The simplest kind of non-rigid transformation is uniform scaling, in which an object changes in size but its angles stay the same. Other non-rigid transformations include stretching, shearing, skewing, and bending, which disrupt both lengths and angles.[3] Both rigid and non-rigid transformations usually result in changes to an object’s 2-D retinal shape. For example, if a square is stretched into an oblong rectangle, this will usually be associated with a change in the shape of its projection on the retina.
Size constancy involves seeing things as sharing/retaining a property across rigid transformations in a retinocentric reference frame (since non-rigid transformations usually change an object’s size). For example, one might perceptually represent something as retaining a particular size property despite viewing it at different distances. Shape constancy, as it is normally introduced, involves seeing things as sharing/retaining a property despite either a rigid transformation (e.g., rotation or translation with respect to the retina and the line of sight), or uniform scaling. For example, one might see an object as retaining a particular distal shape despite viewing it at different orientations (slants) in depth.
Structure constancy cannot be reduced to size or shape constancy. The reason is that structure constancy involves seeing an object as retaining a property (which I’ll label “compositional structure”) across certain non-rigid transformations that (unlike uniform scaling) disrupt both the distances and angles among parts of the object. As such, structure constancy is distinctive insofar as the transformations relevant to exercising structure constancy are different from (and, as we’ll see, more geometrically complicated than) the transformations relevant to exercising the other geometrical constancies.
3. The Visual Phenomenology of Structure Constancy
Many of the most ecologically significant objects with which we interact are biological objects—especially animals and other humans. Many biological objects have an important characteristic: When they move, they change shape. This happens when, for instance, a person walks across a room. Even though the person’s precise metric properties are constantly changing, intuitively we are able to see her body as retaining some important aspects of structure as she moves. In this section I will first introduce the notion of compositional structure. Then I will propose that structure constancy is most plausibly explained by the proposal that visual experience represents compositional structure.
3.1. Compositional structure introduced
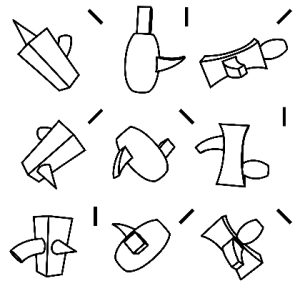
Objects often seem to decompose naturally into parts. For example, the object in figure 1a seems to have three natural parts, as shown in figure 1b.

In addition to being intuitively compelling, judgments about an object’s decomposition into parts are remarkably consistent across observers (e.g., De Winter & Wagemans 2006). This, in addition to its role in several well-known theories of object recognition (Marr & Nishihara 1978; Biederman 1987), has led part decomposition to become a topic of extensive research in perceptual psychology.[4]
Critically, there are rules by which the visual system parses objects into parts. An important rule for our purposes is called the minima rule, first formulated by Hoffman and Richards (1984). The minima rule states that the boundaries between the perceived parts of an object tend to be found at extrema of negative curvature—roughly, places at which the surface of the object is locally most concave. Concave regions are, intuitively, regions where the object’s surface curves “inward.” Figures 2a and 2b illustrate applications of the minima rule in specifying part boundaries.

While the minima rule tells us where to find boundaries between parts, it does not tell us precisely how to “slice” an object. That is, it does not specify how to make part cuts. Fortunately, this problem has also been studied extensively. Other things being equal, part cuts tend to obey the short-cut rule (Singh, Seyranian, & Hoffman 1999), which states that the visual system prefers part cuts that link negative minima of curvature, and generally opts for the shortest such links possible. The part cuts in figure 1b conform to the short-cut rule, as would the most obvious cuts of figures 2a and 2b.[5]
The representation of part decomposition (roughly in accordance with the minima and short-cut rules) has incredible psychological utility (e.g., Ling & Jacobs 2007). For example, many objects that move non-rigidly nevertheless change shape in a systematic manner. Roughly, their parts retain their intrinsic shapes, though the spatial relations between parts may change. The moving human body, as we saw, is an instance of this generalization, but so are the moving bodies of most other animals, along with many manufactured devices (such as, e.g., a stapler or a reclining chair). By decomposing a complex object into parts one can predict the ways it is likely to transform over time. It is disposed to move in ways that alter the spatial relations between parts, but unlikely to move in ways that either alter the intrinsic shapes of parts or displace the joints about which the parts rotate.
We are now ready to introduce the notion of compositional structure. A compositional structure of an object O consists of the following:
- A decomposition of O into a pairwise disjoint set of (proper) parts P1…Pn,
- The approximate part-centered locations of boundaries between connected pairs of parts in P1…Pn,
- The approximate intrinsic shapes of P1…Pn.
Structure constancy amounts, I suggest, to the ability to perceptually represent an object as retaining a particular compositional structure across proximal cue variations (e.g., changes in retinal shape) that result from non-rigid transformations of the object.
A terminological note: A set of parts P1…Pn will be called “pairwise disjoint” if and only if for all pairs (Pi, Pj) drawn from P1…Pn, Pi and Pj do not overlap. Now, three substantive remarks on the visual representation of compositional structure:
First, according to my characterization of compositional structure, an object will have at least as many compositional structures as it has decompositions into parts. This may give rise to some initial concerns. For decompositions are cheap. An object can be decomposed in any number of ways, and it certainly does not seem as though we perceptually experience all of these decompositions, much less perceive them all as remaining stable as an object moves. However, the explanation of structure constancy offered here does not rely on this claim. Rather, the idea is that a particular compositional structure is perceptually represented, while the others are not.
Second, note that I take only the approximate intrinsic shapes of parts to figure in compositional structure. Due to, say, the deformation of muscle tissue, a person’s upper arm does not retain its metric properties precisely as the arm rotates. So it is likely that to perceive an object as retaining compositional structure over time, the object’s parts need only retain their shapes up to some more coarse-grained standards of precision.
Third, note that the locations of part boundaries must be specified in part-centered reference frames. This means that the locations of part boundaries are represented via their spatial relations to certain points on the connected parts themselves. The reason is this: If, say, the location of a perceived person’s elbow (a boundary between forearm and upper arm) is specified in a viewer-centered reference frame, then its location does change as the person moves. Similarly, if its location is specified in a simple object-centered reference frame (e.g., with an origin at the center of gravity of the person’s body), then its location changes as a result of rotation of the upper arm about the shoulder joint. Only when the elbow’s location is specified in a frame of reference centered on either the forearm or upper arm (according to their intrinsic axes) does its location remain approximately stable across nonrigid movement of the body. Like the representation of metric part shapes, the representation of part boundaries should be somewhat coarse-grained. Even in a part-centered reference frame, part boundaries do not remain perfectly stable across non-rigid movement.
3.2. Representing compositional structure in experience
I’ve proposed that the compositional structure of an object is represented in visual experience, and that this is what accounts for the experience of structure constancy. But this claim requires further defense. In what follows I’ll defend it using a modification of Susanna Siegel’s method of phenomenal contrast (Siegel 2010).
Siegel’s method is introduced as a procedure for determining whether visual experiences represent a given property F. It requires us to examine two overall experiences that differ phenomenally, and determine whether the best explanation of their phenomenal contrast is that one of the overall experiences contains a visual experience that represents F, while the other does not.
Unfortunately, Siegel’s method of phenomenal contrast cannot be straightforwardly applied in the current case. Consider any two experiences A and B that phenomenally differ, and are plausible candidates for differing vis-à-vis the compositional structures they represent. The method asks us to determine whether the phenomenal contrast between A and B is best explained by the hypothesis that they indeed differ with respect to the visual experiential representation of compositional structure. However, for any two such experiences, there will plausibly be numerous other differences in their visual experiential content, and some of these other differences would also seem to plausibly explain the phenomenal contrast.
Notice that if an object loses a particular compositional structure, it must cease to occupy precisely the same spatial region. For example, any change in the intrinsic shape of an object O’s part P necessitates a change in O’s compositional structure, but it also necessitates a change in the precise spatial region that O occupies. Thus, if we only consider this individual change, the difference in phenomenology that accompanies successive experiences of O (before and after the change) may seem to be explained just as well by the hypothesis that visual experience only represents the precise spatial region that O occupies, rather than O’s compositional structure.
How should we evaluate the hypothesis that visual experiences represent compositional structure? I suggest that, rather than examining two individual experiences, we ought to examine pairs of changes in experience. We begin with an experience of a base stimulus, and a hypothesis about the particular compositional structure C of the base stimulus represented in experience. Next, we consider the experiences of two test stimuli. Test stimulus 1 shares compositional structure C with the base stimulus, while test stimulus 2 does not. However, both test stimuli differ from the base stimulus in their precise metric structure. If visual experiences represent compositional structure, then one might expect the difference between one’s experiences of the base stimulus and test stimulus 2 to be more salient than the difference between one’s experiences of the base stimulus and test stimulus 1.
However, for this to be a fair test, we need to ensure that, as regards factors besides compositional structure, the change from the base stimulus to test stimulus 1 is either roughly comparable to, or else greater than, the change from the base stimulus to test stimulus 2. In particular, we want to ensure that the increase in salience accompanying the change between the base stimulus and test stimulus 2 is not due to a greater difference in local features of the stimuli, or to a greater “overlap” in their spatial regions.
There are a variety of ways to measure the amount of local point or feature difference between two figures (see, e.g., Kayaert et al. 2003; Veltkamp & Latecki 2006). Perhaps the most straightforward measure is “Hamming distance” (Ullman 1996: 5). To find this distance, we first specify the two figures within a coordinate system. Each is represented by a binary vector indicating, for each point p within the coordinate system, whether p “belongs” to the figure (“1” if it belongs, “0” if it does not). Given this, we measure the distance between the two figures by normalizing the figures to a standard position and orientation, then summing the places in which the vectors for the two figures differ.
In what follows I’ll only consider cases in which the Hamming distance between the base stimulus and test stimulus 1 is clearly either greater than, or roughly comparable to, the Hamming distance between the base stimulus and test stimulus 2. The argument is that if the difference between the base and test stimulus 2 is more phenomenologically salient under these conditions, then the best explanation is that visual experience represents the base stimulus as sharing a property with test stimulus 1, but doesn’t attribute this property to test stimulus 2. My proposal is that the former two are visually experienced as sharing a compositional structure.
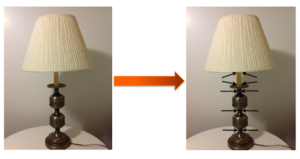
Consider figures 3a-3c. Let 3a serve as our base stimulus. Its compositional structure C plausibly consists of the following: a decomposition into head, torso, arms, and legs; the approximate intrinsic shapes of these parts; and the joints at which they are connected to one another. Figure 3b (test stimulus 1) shares C with the base stimulus. Figure 3c (test stimulus 2) does not share C with the base stimulus (joint locations are changed). Phenomenologically, I find these changes to be qualitatively different. The transformation to 3b seems “natural,” while the transformation to 3c doesn’t, even though the Hamming distance between the base and test stimulus 1 is obviously greater than the distance between the base and test stimulus 2. The proposal that visual experience represents compositional structure explains this. In the first case, the two objects are visually experienced as sharing a feature (a particular compositional structure), while in the second case, they are not.

Consider another example, due to Ling and Jacobs (2007). The base stimulus is shown in figure 4a, while the test stimuli 1 and 2 are shown in figures 4b and 4c, respectively. Again, the Hamming distance between the base and test stimulus 1 is greater (i.e., the two have less overlap in local features), but the transition between the two arguably seems less salient (and also more natural) than the transition from the base to test stimulus 2. Once again, test stimulus 1 preserves compositional structure, while test stimulus 2 does not.
3.3. A post-perceptual explanation?
There are two potential worries with examples involving human bodies, bunny ears, and the like. First, it is unclear whether the contrast in salience here is due to visual experience, or rather to postperceptual expectations given familiarity with such objects and the ways they move. Second, even if the example does reveal the representation of compositional structure in visual experience, it is unclear how general its implications are. Perhaps compositional structure is represented in visual experience only for highly familiar figures, and not for decomposable figures in general. For these reasons, it would be more persuasive if such contrasts in salience could be demonstrated using novel shapes.
Evidence suggests that compositional structure is extracted for novel shapes. Barenholtz and Tarr (2008) showed subjects a novel base shape, along with two transformations of the base shape. Only one of these transformations—which I’ll again label test stimulus 1—preserved compositional structure under the minima and short-cut rules. The shape that failed to preserve compositional structure—test stimulus 2—could involve either a change in location of a boundary between parts, or a change in a part’s intrinsic shape. Figure 5 shows a case in which test stimulus 2 involves a change of the former type. The differences between the base stimulus and test stimuli 1 and 2 are essentially equated in their low-level feature changes, because the narrower part on the right of the figure was rotated the same amount in both cases. The only difference was whether the part’s axis of rotation was its joint with the rest of the object (preserving compositional structure) or its endpoint (altering compositional structure).
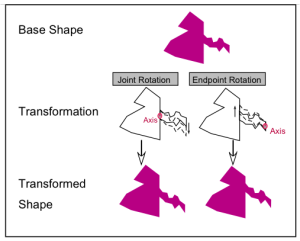
Participants saw the three shapes, and were simply asked to indicate which of the transformed shapes was more similar to the base. Barenholtz and Tarr found that subjects were significantly more likely to indicate that the shape that preserved compositional structure was more similar. The same pattern of results was obtained with other triples of shapes where the change that disrupted compositional structure instead altered the intrinsic shape of the base stimulus’s part, rather than its joint location. Thus, there is evidence that the ability to extract compositional structure is highly general and not limited to particular classes of familiar objects (e.g., human or animal shapes).
Nevertheless, how do we know that compositional structure isn’t recovered post-perceptually, even in the case of novel objects? If this were the case, then structure constancy wouldn’t really deserve to be labeled a perceptual constancy at all. Although it is difficult to settle the matter conclusively, there is empirical evidence that the representation of compositional structure is genuinely perceptual.
I’ve held that the explanation of structure constancy resides in the visual system’s ability to decompose objects into parts and represent their boundaries and shapes independently. Because of this, visual experience distinguishes transformations that preserve a given compositional structure from those that do not. As such, evidence for the perceptual differentiation of parts provides support for the view that structure constancy is perceptual.
Perhaps the strongest evidence that parts are extracted perceptually is that part decomposition influences other paradigmatically perceptual processes. A striking example of this involves the perception of transparency. Compare figures 6a and 6b.

Figures 6a (left) and 6b (right). Source: Singh & Hoffman (2001)
While figure 6a appears to depict a transparent gray filter in front of a half-dark, half-light background, in figure 6b the percept of transparency is greatly diminished (Singh & Hoffman 1998). Rather, the occluding object is perceived as an opaque figure with two differently shaded regions. The received explanation for this is that the visual system expects regions of a single part of an object to have the same reflectance, but it does not expect regions of different parts of an object to have the same reflectance (or at least it expects this less strongly). Since the object in figure 6b can be broken down into two natural parts, it can be interpreted as an opaque figure whose parts have different reflectances.[6]
If part decomposition interacts with other perceptual processes, we have strong evidence that it is a perceptual process as well. For, while it is possible to advert to a cognitive penetration account in these cases, I can think of no motivation for doing so (aside from a pretheoretical conviction that part decomposition must be cognitive). Moreover, it is worth noting that the tendency to parse objects into parts also strikes me as involuntary—I cannot help seeing many objects as decomposed into natural parts. This is another hallmark feature of a perceptual process (e.g., Fodor 1983; Pylyshyn 1999).[7]
Even if parts are represented during perception, this does not yet show conclusively that compositional structure is represented during perception. The representation of compositional structure involves both decomposing an object into parts and (i) representing the intrinsic shape of each part independently, and (ii) representing the part-centered boundaries between parts.
With respect to (i), there are good reasons to believe that the visual system encodes the shapes of different parts separately from one another, and independently of their spatial relations. Though this hypothesis was initially put forth on computational and theoretical grounds (e.g., Biederman 1987; Marr & Nishihara 1978; Palmer 1978), there is now compelling experimental evidence for it. Consider a recent study of the subject S.M., an individual with integrative agnosia. Integrative agnosia is a visual disorder that affects processes involving the integration of local visual information into a global percept. Behrmann et al. (2006) found that S.M. was capable of correctly discriminating sequentially presented objects from one another when the objects differed in the intrinsic shape of a single part (e.g., a cube-shaped part versus an ellipsoid-shaped part), but, unlike “normal” participants, he could not discriminate objects when they differed purely in their parts’ spatial configuration (e.g., a cube to the left of a cylinder versus a cube on top of a cylinder). In line with (i), this suggests that there are visual processes that extract the shapes of individual parts, and these processes can remain intact despite an inability to extract the global configuration of an object (see also Davidoff & Roberson 2002; Cacciamani, Ayars, & Peterson 2014). Again, absent defeating evidence, I conclude that the processing of individual part shapes happens within perception.
The claim, (ii), that part boundaries are represented in part-centered reference frames is the hardest to establish. Before covering empirical support for this claim, we need to get clearer on what part-centered reference frames are, and how they have been developed in the vision science literature.
Constructing a reference frame involves choosing a set of parameters so that the position of any point is uniquely determined by specifying its values on these parameters (Klatzky 1998). When a reference frame is centered on an object O, this means that the positions of points are encoded at least partly in terms of their spatial relations (e.g., distance and direction) to a point, or set of points, on O. For example, to construct a polar coordinate system, we first stipulate an origin o and an axis A through o, and then specify the location of any given point p in terms of two parameters: its distance from o, and the angle between A and the line from o to p.
Many shape representation theorists have proposed that the visual system recovers, roughly, the medial axis structure of an object (e.g., Blum & Nagel 1978; Rosenfeld 1986; Kimia 2003; Feldman & Singh 2006). The medial axis of a figure is composed of the set of points having two or more closest points on the bounding contour of the figure. A figure’s medial axis generally looks like a “skeleton” from which the figure is “grown.” These schemes are centered on the points that compose the axis. Roughly, they represent the positions of points on the boundary of the shape by specifying their distances and directions from corresponding points on the axis.
Importantly, in a wide range of cases, the medial axis structure of an object bears a close relation to its decomposition into parts under the minima and short-cut rules.[8] This is because different parts of the object tend to be associated with distinct axis branches (see figure 7). Thus, if the visual system extracts the medial axis structures of objects, and distinct parts are associated with distinct axis branches, then these distinct axis branches can be used to construct separate reference frames each centered on a distinct part. Accordingly, evidence for the visual representation of medial axis structure also counts as evidence that the visual system uses part-centered frames of reference.

The prediction that vision extracts medial axis structure has recently been confirmed using a very simple paradigm. Firestone and Scholl (2014) showed subjects a novel shape, asked them to tap the shape wherever they liked, and recorded the locations of subjects’ taps. If “tapping” behavior is guided by a visual shape representation that specifies the intrinsic (perhaps medial) axes of object parts, one might expect the locations of subjects’ taps to be influenced by these axes. Sure enough, Firestone and Scholl found that the recorded taps (when aggregated) corresponded closely to the medial axes of the shapes presented. That is, subjects were much more likely to tap an object somewhere along its medial axis than they were to tap other regions of the shape. This provides compelling evidence that medial axis structure is automatically extracted by vision, since the task did not require subjects to attempt to extract these axes.
If the visual system represents spatial properties and relations by using intrinsic part axes, then it should encode the parts of an object as retaining their spatial relations to one another across transformations in viewer-centered position and orientation. For as long as these transformations are rigid, the part-centered relations between the constituents of the configuration will not change.
There is intriguing evidence that areas of the visual system code for medial axis structure independently of viewer-centered position. In a recent fMRI study, Lescroart and Biederman (2013) presented subjects with figures that differed in either their medial axis configuration, their component part shapes, or their viewer-centered orientation. Figure 8 displays some of these figures: Shapes in the same row share the same medial axis structure, though their orientations and intrinsic part shapes vary. Stimuli in the same column share the same intrinsic part shapes, but differ in medial axis structure. The line segments next to the figures indicate viewer-centered orientation. Lescroart and Biederman found that by area V3, patterns of BOLD activity could classify stimuli according to shared medial axis structure at a rate significantly better than chance (even though such stimuli differed in the shapes of their component parts), and classification of medial axis structure was significantly more accurate than classification of orientation (whereas the opposite pattern was observed in V1). This provides at least prima facie evidence that extrastriate areas of the visual system represent configurations according to spatial arrangements of part axes.
We have encountered strong evidence that each of the characteristics that figure in compositional structure is recovered during vision proper. I contend that the proposal that compositional structure is represented in subpersonal visual processing and manifests itself in visual experience offers the best account in light of all the evidence at our disposal, including both the patterns of phenomenological salience associated with shape transformations in objects, and the empirical data on subpersonal shape processing.
4. Mereological Structure and Shape Representation Schemes
What does structure constancy tell us about the subpersonal underpinnings of shape experience? I believe it has at least two important consequences for these underpinnings. In this section, I’ll argue that structure constancy has the consequence that certain aspects of shape experience must be underpinned by a representation scheme that is mereologically structured. In the next, I’ll argue that because structure constancy must recruit non-viewer-centered reference frames, it raises problems for recent approaches on which spatial phenomenology is subserved by some enrichment of Marr’s 2½-D sketch.
Call a representation R mereologically structured iff:
- R purports to introduce individuals O and O* independently, and
- R represents that O is a proper part of O*.
To purport to introduce n individuals independently is to deploy n distinct representational items that each purport to introduce distinct individuals.[9] For instance, the phrases “John’s cat” and “John’s dog” purport to introduce two individuals independently. The central idea, then, is that if a representation R is mereologically structured, then distinct constituents of R purport to pick out distinct entities that are related through mereological composition, and R represents the parthood relations that those entities stand in to one another.
Some shape representation schemes are not mereologically structured. Consider, for instance, schemes found in the view-based approach to object recognition (see, e.g., Ullman and Basri 1991; Ullman 1996; Edelman 1999; Riesenhuber & Poggio 2002). On several of these models, the representation of shape just amounts to the representation of a vector composed of the viewer-centered feature coordinates of some of the object’s “critical features”—e.g., vertices, inflection points, and curvature maxima.[10] This type of scheme does not incorporate the representation of parthood at all, and proponents of the view-based approach have often downplayed the role of part decomposition in visual processing (e.g., Edelman 1999: 89-94).
Perhaps the most popular mereologically structured scheme is hierarchical description (see, e.g., Palmer 1977; Marr & Nishihara 1978; Feldman 2003; Leek et al. 2009; Hummel 2013). A hierarchical description (figure 9) is a representational structure that contains distinct nodes corresponding to each individual introduced, encodes either mereological or spatial relations between nodes, and associates monadic featural information with each node. It is usually depicted as a tree.
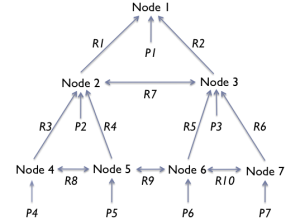
Edges traversing levels of a hierarchical description represent parthood. For present purposes, I’ll assume that the visual system’s representation of parthood is transitive: If a hierarchical description represents O1 as part of O2 and O2 as part of O3, then it also represents O1 as part of O3. Edges linking nodes at the same level of a description represent spatial relations between parts. Let’s call edges representing parthood P-edges, and edges representing spatial relations S-edges. A subset of the S-edges will describe the locations of boundaries between parts: They will represent, for a pair of connecting parts, the points where those parts meet (in part-centered coordinates). Call these B-edges.
Several models of shape processing invoke both an earlier, view-based stage and a later, hierarchical stage (Marr 1982; Hummel 2001, 2013). If this is right, it is natural to ask which (if either) of these stages underpins shape phenomenology. I argue that structure constancy provides strong reason to locate at least certain aspects of shape phenomenology at the hierarchical stage.
It is hard to see how a view-based scheme could underpin structure constancy. Because view-based schemes do not introduce the parts of objects as distinct individuals, such models do not prioritize any particular part decomposition over others. Each of the many possible decompositions of an object into parts is compatible with, say, the same arrangement of vertices and curvature extrema along the object’s bounding contour. Because view-based schemes fail to prioritize a specific part decomposition, they lack the resources for distinguishing changes that leave intrinsic part shapes intact while altering the global shape of the object from changes that alter the intrinsic shapes of parts. Indeed, any given change could—relative to some decomposition—be considered a change in the intrinsic shapes of an object’s parts. Thus, without a specification of which decomposition is the relevant one, it is impossible to decide whether a particular change does or does not deform intrinsic part shapes.
Hierarchical description, on the other hand, can be applied to the explanation of structure constancy. Let’s spell this out using the human body as an example. Given a hierarchical description that introduces a human body O, a compositional structure of O is encoded in (i) the intrinsic shape information associated with nodes at some level of the description lower than the level at which O is introduced, such as a level introducing the head, torso, arms, and legs, (ii) the P-edges linking these nodes to the node introducing O, and (iii) the B-edges linking these nodes to one another—e.g., torso-centered locations of the shoulders, where the arms intersect the torso. By distinguishing this information from the information encoded by the remaining S-edges (such as the angle formed between an arm and the torso) and information about O’s global metric structure, the representation enables the visual system to distinguish transformations that leave a given compositional structure intact from those that do not. As such, hierarchical descriptions may underpin structure constancy.
5. Comparison with Other Approaches
Many have been attracted to the idea that visual phenomenology seems to present us with an array of facing surfaces, rather than, e.g., the 2-D retinal image or the volumetric structure of objects (e.g., Jackendoff 1987; Tye 1991, 1995; Prinz 2012). In light of this, theorists influenced by Marr’s (1982) pioneering tripartite theory of vision have sought to locate the underpinnings of visual consciousness at the “intermediate” level of processing, which describes the geometry of surfaces. In Marr’s framework, the intermediate level is occupied by the 2½-D sketch, so theorists have often appealed to the 2½-D sketch, though usually with some alterations or enrichments, which I’ll discuss below.
The 2½-D sketch is an array specifying the viewer-centered distance, direction, and local orientation at each point (up to a certain resolution) for all visible surfaces in the scene (see Marr 1982: 275-279). It can be construed as a type of “depth map” representing certain spatial properties of thousands of very small surface patches within one’s field of vision. The important thing to note is that the 2½-D sketch lacks two features that I have argued are central to explaining structure constancy. First, the scheme is mereologically unstructured. This is because the 2½-D sketch only attributes geometrical features to very small surface patches in one’s field of vision, and it does not represent the composition of such surface patches into larger individuals. Second, the scheme is wholly viewer-centered. That is, all locations in the visual field are represented relative to an origin centered on the viewer. So the locations of part boundaries are not represented in part-centered coordinates.
Jackendoff (1987) calls on the 2½-D sketch in his account of the subpersonal underpinnings of conscious experience, but recognizes that Marr’s representational structure has important defects (e.g., lack of explicit surface segmentation, perceptual grouping, etc.). As such, he develops an enriched 2½-D sketch, which he calls the 2½-D structural description (see Jackendoff 1987: 331-338). More recently, Prinz (2012) has appealed to Jackendoff’s theory in his “intermediate view” of the subpersonal basis of visual consciousness.
Jackendoff enriches Marr’s depth map with the primitive elements boundary and region, and the predicates directed, abutting, overflow, and occlusion. Boundaries and regions are obtained by appropriately segmenting the initially undifferentiated 2½-D sketch. The predicates represent properties and relations of these boundaries and regions. For example, the 2½-D structural description has the resources to encode (via the directedness predicate) figure-ground relations, and can encode (via the overflow predicate) that a region extends outside one’s field of vision. Moreover, Jackendoff also incorporates parthood into his 2½-D structural description. He proposes that boundaries are identified not only where one finds luminance edges in the retinal image, but also in accordance with Hoffman and Richards’ minima rule.
For our purposes, the important point is this. Jackendoff’s model organizes the visual array into objects and parts, but it does not alter the basic reference frame of the 2½-D sketch. The depth map is segmented, and certain properties of segmented regions are represented, but the underlying coordinate frame remains wholly viewer-centered. Likewise, although Prinz (2012) offers some revisions to Jackendoff’s model, he agrees that the representation underlying visual consciousness is wholly viewer-centered (Prinz 2012: 50-57). See also Tye (1991: 90-97; 1995: 140-141) for a similar view.
For the reasons canvassed above, viewer-centered representational schemes cannot plausibly underpin structure constancy. Whenever an object moves relative to the perceiver, the viewer-centered locations of its part boundaries change. But to explain the patterns of phenomenological salience associated with shape transformations, we need a representation that treats part boundaries as remaining stable across such changes in viewer-centered location, so long as they don’t shift their positions relative to the connected parts themselves. A part-centered scheme does this, while a viewer-centered scheme does not.
As such, the view I have offered importantly departs from these approaches on a critical dimension of shape representation (viz., its reference frame), though it does have a feature in common with them (viz., incorporating part-based organization).
I should underscore, however, that the view that the locations of certain things are experienced in part-centered reference frames does not imply—or even suggest—that we fail to also experience things in a viewer-centered reference frame. Indeed, it is an undeniable aspect of our phenomenology that we perceive from a perspective, e.g., that objects are seen to have certain spatial relations to our point of view (e.g., Peacocke 1992; Schellenberg 2008; Bennett 2009). Nevertheless, I think that on the most plausible analysis, vision represents geometrical/spatial properties within multiple reference frames simultaneously (cf. Briscoe 2009; Humphreys et al. 2013). Indeed, the view that perception uses multiple reference frames seems to comport best with the overall phenomenology of watching a non-rigid object move. When a person walks, for example, there is a sense in which her joint locations seem to stay stationary, but also a sense in which they seem to move relative to your viewpoint.
6. Conclusion
In this paper I have offered an account of a novel type of geometrical constancy, which I’ve called structure constancy. I argued that we visually experience objects as retaining their compositional structure despite certain changes that alter their intrinsic metric properties. Moreover, I have drawn out implications of structure constancy for both the representational content and the subpersonal underpinnings of visual shape experience.
References
Bar, M. (2001). Viewpoint dependency in visual object recognition does not necessarily imply viewer-centered representation. Journal of Cognitive Neuroscience, 13, 793-799.
Barenholtz, E., & Feldman, J. (2003). Visual comparisons within and between object parts:Evidence for a single-part superiority effect. Vision Research, 43, 1655-1666.
Barenholtz, E., & Tarr, M.J. (2008). Visual judgment of similarity across shape transformations:Evidence for a compositional model of articulated objects. Acta Psychologica, 128, 331-338.
Behrmann, M., Peterson, M.A., Moscovitch, M., & Suzuki, S. (2006). Independent representation of parts and the relations between them: Evidence from integrative agnosia. Journal of Experimental Psychology: Human Perception and Performance, 32(5), 1169-1184.
Bennett, D.J. (2009). Varieties of visual perspectives. Philosophical Psychology, 22(3), 329-352.
Bhatt, R.S., Hayden, A., Kangas, A., Zieber, N., & Joseph, J.E. (2010). Part perception in infancy: Sensitivity to the short-cut rule. Attention, Perception, & Psychophysics, 72(4), 1070-1078.
Biederman, I. (1987). Recognition-by-components: A theory of human image understanding. Psychological Review, 94(2), 115-117.
Blum, H. & Nagel, R. N. (1978). Shape description using weighted symmetric axis features. Pattern Recognition, 10(3), 167-180.
Briscoe, R. (2009). Egocentric spatial representation in action and perception. Philosophy and Phenomenological Research, 79, 423-460.
Burge, T. (2010). Origins of Objectivity. Oxford: Oxford University Press.
Cacciamani, L., Ayars, A.A., & Peterson, M.A. (2014). Spatially rearranged object parts can facilitate perception of intact whole objects. Frontiers in Psychology, 5, 1-11.
Cohen, J. (forthcoming). Perceptual constancy. To appear in M. Matthen (ed.), Oxford Handbook of Philosophy of Perception. Oxford: Oxford University Press.
Davidoff, J., & Roberson, D. (2002). Development of animal recognition: A difference between parts and wholes. Journal of Experimental Child Psychology, 81, 217-234.
DeWinter, J., & Wagemans, J. (2006). Segmentation of object outlines into parts: A large-scale, integrative study. Cognition, 99, 275–325.
Edelman, S. (1997). Computational theories of object recognition. Trends in Cognitive Sciences, 1, 296-304.
Edelman, S. (1999). Representation and Recognition in Vision. Cambridge, MA: MIT Press.
Feldman, J. (2003). What is a visual object? Trends in Cognitive Sciences, 7(6), 252-256.
Feldman, J., & Singh, M. (2006). Bayesian estimation of the shape skeleton. Proceedings of the National Academy of Sciences, 103(47), 18014-18019.
Feldman, J., Singh, M., Briscoe, E., Froyen, V., Kim, S., & Wilder, J. (2013). An integrated Bayesian approach to shape representation and perceptual organization. In S.J. Dickinson & Z. Pizlo (eds.), Shape Perception in Human and Computer Vision, pp. 55-70. New York: Springer.
Firestone, C., & Scholl, B.J. (2014). “Please tap the shape, anywhere you like”: Shape skeletons in human vision revealed by an exceedingly simple measure. Psychological Science, DOI: 10.1177/0956797613507584.
Fodor, J.A. (1983). Modularity of Mind. Cambridge, MA: MIT Press.
Graf, M. (2006). Coordinate transformations in object recognition. Psychological Bulletin, 132, 920-945.
Hill, C.S. (2014). The content of visual experience. In Meaning, Mind, and Knowledge, pp. 218-Oxford: Oxford University Press.
Hoffman, D.D. (2001). Mereology of visual form. In C. Arcelli, L.P. Cordella, & G.S. di Baja (eds.), Visual Form 2001, pp. 40-50. New York: Springer.
Hoffman, D.D., & Richards, W.A. (1984). Parts of recognition. Cognition, 18, 65-96.
Hoffman, D.D., & Singh, M. (1997). Salience of visual parts. Cognition, 63, 29-78.
Hummel, J. E. (2001). Complementary solutions to the binding problem in vision: Implications for shape perception and object recognition. Visual Cognition, 8, 489-517.
Hummel, J.E. (2013). Object recognition. In D. Reisburg (ed.), Oxford Handbook of Cognitive Psychology, pp. 32-46. Oxford: Oxford University Press.
Humphreys, G., Gillebert, C.R., Chechlacz, M., & Riddoch, M.J. (2013). Reference frames in visual selection. Annals of the New York Academy of Sciences, 1296, 75-87.
Jackendoff, R. (1987). Consciousness and the Computational Mind. Cambridge, MA: MIT Press.
Kayaert, G., Biederman, I., & Vogels, R. (2003). Shape tuning in macaque inferior temporal cortex. The Journal of Neuroscience, 23, 3016–27.
Kimia, B.B. (2003). On the role of medial geometry in human vision. Journal of Physiology- Paris, 97, 155-190.
Klatzky, R.L. (1998). Allocentric and egocentric spatial representations: Definitions, distinctions, and interconnections. In C. Freksa, C. Habel, & K.F. Wender (eds.), Spatial Cognition, pp. 1-17. Berlin: Springer.
Leek, E.C., Reppa, I., Rodriguez, E., & Arguin, M. (2009). Surface but not volumetric part structure mediates three-dimensional shape representation: Evidence from part–whole priming. The Quarterly Journal of Experimental Psychology, 62(4), 814-830.
Lescroart, M.D., & Biederman, I. (2013). Cortical representation of medial axis structure. Cerebral Cortex, 23, 629-637.
Ling, H., & Jacobs, D. W. (2007). Shape classification using the inner-distance. IEEE Transactions on Pattern Analysis and Machine Intelligence, 29(2), 286-299.
Marr, D. (1982). Vision: A Computational Investigation into the Human Representation and Processing of Visual Information. San Francisco: W.H. Freeman.
Marr, D., & Nishihara, H. K. (1978). Representation and recognition of the spatial organization of three-dimensional shapes. Proceedings of the Royal Society of London, B: Biological Sciences, 200, 269–294.
Michaels, C.F., & Carello, C. (1981). Direct Perception. Englewood Cliffs, NJ: Prentice-Hall.
Noë, A. (2004). Action in Perception. Cambridge, MA: MIT Press.
Palmer, S.E. (1977). Hierarchical Structure in Perceptual Representation. Cognitive Psychology, 9, 441-474.
Palmer, S.E. (1978). Fundamental aspects of cognitive representation. In E. Rosch & B. Lloyd (eds.), Cognition and Categorization, pp. 261-304. Hillsdale, NJ: Lawrence Erlbaum.
Palmer, S.E. (1999). Vision Science: Photons to Phenomenology. Cambridge, MA: MIT Press.
Peacocke, C. (1992). A Study of Concepts. Cambridge, MA: MIT Press.
Pizlo, Z. (2008). 3D Shape: Its Unique Place in Visual Perception. Cambridge, MA: MIT Press.
Prinz, J.J. (2012). The Conscious Brain: How Attention Engenders Experience. Oxford: Oxford University Press.
Pylyshyn, Z.W. (1999). Is vision continuous with cognition? The case for cognitive impenetrability of visual perception. Behavioral and Brain Sciences, 22, 341-423.
Pylyshyn, Z.W. (2007). Things and Places: How the Mind Connects with the World. Cambridge, MA: MIT Press.
Recanati (2012). Mental Files. Oxford: Oxford University Press.
Riesenhuber, M. & Poggio, T. (2002). Neural mechanisms of object recognition. Current Opinion in Neurobiology, 12, 162-8.
Rock, I. (1983). The Logic of Perception. Cambridge, MA: MIT Press.
Rosenfeld, A. (1986). Axial representations of shape. Computer Vision, Graphics, and Image Processing, 33(2), 156-173.
Schellenberg, S. (2008). The situation-dependency of perception. The Journal of Philosophy, 105, 55-84.
Shoemaker, S. (2000). Introspection and phenomenal character. Philosophical Topics, 28, 247-273.
Siegel, S. (2010). The Contents of Visual Experience. Oxford: Oxford University Press.
Singh, M. & Hoffman, D. D. (1998). Part boundaries alter the perception of transparency. Psychological Science, 9, 370-378.
Singh, M., & Hoffman, D.D. (2001). Part-based Representations of Visual Shape and Implications for Visual Cognition. In T.F. Shipley & P.J. Kellman (eds.), From Fragments to Objects: Segmentation and Grouping in Vision. New York, NY: Elsevier Science.
Singh, M., Seyranian, G.D., & Hoffman, D.D. (1999). Parsing silhouettes: The short-cut rule. Perception & Psychophysics, 61, 636-660.
Smith, A.D. (2002). The Problem of Perception. Cambridge, MA: Harvard University Press.
Tye, M. (1991). The Imagery Debate. Cambridge, MA: MIT Press.
Tye, M. (1995). Ten Problems of Consciousness: A Representational Theory of the Phenomenal Mind. Cambridge, MA: MIT Press.
Ullman, S. (1996). High-Level Vision: Object Recognition and Visual Cognition. Cambridge, MA: MIT Press.
Ullman, S. & Basri, R. (1991). Recognition by linear combinations of models. IEEE Transactions on Pattern Analysis & Machine Intelligence, 13, 992-1006.
Veltkamp, R.C. & Latecki, L.J. (2006). Properties and performance of shape similarity measures.In Proceedings of the 10th IFCS Conference on Data Science and Classification, Slovenia, July 2006.
Xu, Y., & Singh, M. (2002). Early computation of part structure: Evidence from visual search. Perception & Psychophysics, 64(7), 1039-1054.
Notes
[1] Roughly this notion appears in the work of a variety of authors, such as Michaels and Carello (1981: 20), Rock (1983: 24), Smith (2002), and Pizlo (2008). Other notions of constancy instead focus on the stability of one’s perceptual representation across changes in a property’s appearance (e.g., Shoemaker 2000; Noë 2004; Hill 2014).
[2] Notice that these changes may be either intra-object or inter-object: a perceiver may continue to perceive the same object as P despite changes in the proximal stimulation received from it, or a perceiver may perceive different objects as both being P despite differences in the proximal stimulation received from them.
[3] In geometry, transformations are arranged into groups, such as affine transformations, projective transformations, and topological transformations. The group consisting only of rigid transformations and uniform scaling is the similarity group. Often, the similarity group is taken to be definitive of what we mean when we say that two objects have the “same shape.” We mean that one can be brought into precise register with the other by some composition of similarity transformations (Palmer 1999: 364-365).
[4] For general discussions, see Singh and Hoffman (2001), and Hoffman (2001).
[5] However, these rules have exceptions. See Singh and Hoffman (2001) for discussion.
[6] Part perception has also been argued inter alia to influence figure-ground organization (Hoffman & Singh 1997), the spread of visual attention (Barenholtz & Feldman 2003) and pop-out effects in visual search (Xu & Singh 2002).
[7] Further evidence for the automaticity of part decomposition is provided by studies of human infants. Using a dishabituation paradigm, Bhatt et al. (2010) have provided compelling evidence that 6 ½ month-old infants are sensitive to the minima and short-cut rules.
[8] In practice, however, the correspondence is not perfect. In standard models (e.g., Blum & Nagel 1978), small perturbations of a shape’s contour give rise to “spurious” axis branches that do not intuitively correspond to distinct parts of the shape. Feldman and Singh (2006) have recently developed a novel, Bayesian approach to axial description that “cleans up” the medial axis representation. The axes returned by their model are not medial axes, although for smooth shapes without many perturbations their axes closely resemble medial axes. Feldman and Singh’s model builds in a prior favoring smoother axes with fewer branches, and its results tend to better match intuitive part cuts.
[9] The notion of introducing an individual is left deliberately vague. For present purposes, it does not matter whether individuals are introduced in vision by description or by singular reference. But for recent defenses of the latter view, see Pylyshyn (2007) and Recanati (2012).
[10] Such views have been offered primarily in order to account for findings indicating that object recognition is sensitive to viewpoint. Such results have sometimes been believed problematic for hierarchical approaches to shape representation, which generally invoke non-viewer-centered reference frames. However, for an argument that hierarchical models can accommodate viewpoint effects on recognition, see Bar (2001).

Structure in Shape Perception
I am a fan of Green’s paper. It is a bold proposal, well supported by the empirical literature. Green’s is a proposal not unlike Marr’s (1982) and Biederman’s (1987), although perhaps a bit more detailed and sophisticated. And, of course, it is couched in terms of phenomenology rather than the psychology of mental representation. But we shall forgive him this detail.
Green’s emphasis on structure constancy, as opposed to the more traditional shape constancy, is an important contribution to the field’s thinking on the problem of shape perception. His emphasis is on the distal stimulus, rather than the proximal stimulus. I think this is a move in the right direction.
And it makes Green’s proposal a delightful move away from the “view-based” approach to pattern recognition that has been so popular in the vision literature of late. In sharp contrast to that approach, Green is Old School: He is considering the Problem to be solved and the manner in which a visual system might solve it. The modern approach, by contrast, is far too motivated by findings from monkey neurophysiology: Start with data about how monkey brains perceive things and work from there. This is a great strategy to the extent that humans are like monkeys. (Not that I have anything against monkeys, but the human brain is unlike a monkey brain in a lot of important respects. For one thing, we humans control, and destroy, the world. Monkeys, not so much.)
Green’s proposal is perhaps even better than he realizes. He did an excellent job summarizing the literature supporting it, but he missed some bits. There is old work by Biederman & Cooper (1991a, b, 1992), Biederman & Gerhardstein (1993, 1995) and C. B. Green & Hummel (1996) that he missed. These papers help to shore-up his proposal that object parts are a critical part of the representation of shape. But there is undoubtedly work I am missing as well.
Green seems concerned about the segmentation problem – and by the problem of representing all pair-wise relations between an object’s parts – in parts-based representations of shape. This is a reasonable concern, pointed out by, among others, Ullman (1996). But I hope to allay his concerns a bit. Assuming a stable parts decomposition (e.g., at matched concavities, as proposed by Green), this “problem” is solved by representing (1) parts only in relation to the other parts to which they are connected (a solution at which Green’s proposal at least hints; see also Saiki & Hummel, 1996, for empirical support for this approach) and (2) representing part-relation conjunctions in a format representing a set (which does not specify an ordering among its elements) rather as a list or vector (both of which do). The latter works quite well and is very naturally captured in a neural computing architecture that uses time (e.g., synchrony of firing) rather than space (i.e., conjunctive coding: which neurons are firing) to represent part-relation bindings. Even in the worst case, when a stable part decomposition cannot be assumed, the compositional approach can fail catastrophically as long as it is not the only route to shape perception and object recognition (Hummel, 2001; Hummel & Stankiewicz, 1996).
Of course, I do have a few quibbles with the paper. It is a mistake to call part segmentation “involuntary” (it requires attention, e.g., Thoma, Hummel & Davidoff, 2004). And Green may underestimate the similarity between his approach and previous approaches (such as those of Marr and Biederman). But overall, Green’s proposal has a great deal of merit.
References
Biederman, I. & Cooper, E. E. (1991a). Priming contour deleted images: Evidence for intermediate representations in visual object recognition. Cognitive Psychology, 23, 393-419.
Biederman, I., & Cooper, E. E. (1991b). Evidence for complete translational and reflectional invariance in visual object priming. Perception, 20, 585-593.
Biederman, I., & Cooper, E. E. (1992). Size invariance in visual object priming. Journal of Experimental Psychology: Human Perception and Performance, 18, 121-133.
Biederman, I., & Gerhardstein, P. C. (1993). Recognizing depth-rotated objects: Evidence and conditions for 3-dimensional viewpoint invariance. Journal of Experimental Psychology: Human Perception and Performance, 19, 1162-1182.
Biederman, I. & Gerhardstein, P. C. (1995). Viewpoint-dependent mechanisms in visual object recognition: A critical analysis. Journal of Experimental Psychology: Human Perception and Performance., 21, 1506-1514.
Green, C. B., & Hummel, J. E. (2006). Familiar interacting object pairs are perceptually grouped. Journal of Experimental Psychology: Human Perception and Performance, 32 (5), 1107-1119.
Hummel, J. E. (2001). Complementary solutions to the binding problem in vision: Implications for shape perception and object recognition. Visual Cognition, 8, 489 – 517.
Hummel, J. E., & Stankiewicz, B. J. (1996). An architecture for rapid, hierarchical structural description. In T. Inui & J. McClelland (Eds.). Attention and Performance XVI: Information Integration in Perception and Communication (pp. 93-121). Cambridge, MA: MIT Press.
Marr, D. (1982). Vision. Freeman: San Francisco.
Thoma, V., Hummel, J. E., & Davidoff, J. (2004). Evidence for holistic representations of ignored images and analytic representations of attended images. Journal of Experimental Psychology: Human Perception and Performance, 30, 257-267.
Ullman, S. (1996). High-level Vision: Object Recognition and Visual Cognition. Cambridge MA: MIT Press.
THE SYNTACTIC STRUCTURE OF SEEING STRUCTURE
1. Perceptual syntax and semantics
The jointly scientific and philosophical project of characterizing perceptual processes as computational operations that generate contentful representations of the distal environment, though still dominant, has come under attack (see, e.g., Martin 2006, Brewer 2011). While a small ocean of ink has been spilled to defend the representationalist framework on a priori grounds, appealing to idealized hallucinations and illusions, by far the most effective method of defense is simply to develop a plausible and empirically substantive account of some feature of perceptual processing and phenomenology in which contentful representations figure as central posits. Green’s paper exemplifies this approach, isolating an element of perceptual phenomenology and providing a thorough characterization of it that appeals ineliminably to conscious perceptual representations. In doing so, he illustrates the explanatory power and flexibility of the representationalist framework.
Green’s core arguments that structural constancies are real features of the visual system and that their representational outputs are constituents of conscious visual experience are broadly convincing. He also develops a positive proposal about the contents of those outputs, which contains (inter alia) the following four claims: (i) they represent the compositional structures of perceived objects and their parts; (ii) they constrain a particular decomposition into parts rather than leaving the proper decomposition unspecified; (iii) they represent parts as standing in hierarchical spatial relations; and (iv) they represent those spatial relations in a “part-centered” spatial framework rather than an egocentric, viewpoint-dependent one.
In what follows, I’ll take (i)–(iv) for granted. As I read Green, he is primarily interested in specifying the semantic properties of the relevant representations—i.e., their contents (see below)—rather than their syntactic properties. My interest in this commentary is what constraints, if any, Green’s semantic analysis places on the syntactic structures of the representations that possess the relevant contents. In particular, I’m curious whether the phenomenon of structure constancy is consistent with a picture on which perceptual representations are wholly iconic (i.e., picture-like), or whether it requires discursive (i.e., sentence-like) representations, or even some other type of syntactic structure entirely. Aside from its intrinsic interest, this matters not only because different syntactic structures will require different forms of computations to operate over it, but also because some theorists (e.g., Carey 2009; Burge 2010, 2014; Block 2014) suggest that the border between perception and cognition partly consists in the fact that percepts are iconic while thoughts are discursive. If hierarchical descriptions are both perceptual and non-iconic, therefore, then his account is incompatible with such ways of understanding mental architecture.
First, some terminological ground-clearing. By ‘semantic properties’, I mean the properties of a representation that are relevant to making the representation about whatever it is about. I assume that the semantic properties of a representation determine or are identical to its contents, which may be understood as Fregean abstracta, as the concrete or abstract targets of the representation, as its accuracy conditions, or in some other way. By ‘syntactic structure’, I mean the way in which the parts of the representational vehicle—the state itself, not its contents—are ordered. I assume that thoughts, for instance, are complex propositional structures composed out of mental representations (concepts) that are connected in virtue of the syntactic (canonically, predicative) structure of the whole thought.
Iconic representations, such as unlabelled photographs and, controversially, mental images (Kosslyn 1994), obey the following principle:
ICONICITY: Every part of an icon that represents X represents some part of X.1
Contrast a photograph of Barack Obama wearing a suit (Fig. 1) with the sentence
(1) Barack Obama is wearing a suit.
A part of Figure 1 represents Obama’s eyebrow, another part represents his left hand, and so on. No part of (1), by contrast, represents any part of Obama; the expression ‘Barack Obama’ is simply used to refer to the man without representing any of his parts.
Figure 1—Obama wearing a suit
Because every part of an icon represents some part of the represented scene, an icon can be segmented however one likes, and every segmented portion will represent some correspondingly segmented portion of the scene. In Figure 2, for example, the picture from Figure 1 has been segmented into two arbitrary parts, A and B. Despite how arbitrary the division is, part A represents part of Obama’s face, part of his tie, part of the flag in the background, and so on.
Figure 2—Obama segmented
Again, contrast (1). We might slice (1) up, but the part ‘Barack…a suit’ doesn’t represent anything, nor does part of ‘Barack Obama’ (e.g., ‘ck Oba’). Discursive representations like (1) thus have canonical decompositions into privileged parts (Fodor 2007). In the case of (1), it should be carved into ‘Barack Obama’, ‘is’, ‘wearing a suit’, which may then be carved into ‘wearing’, ‘a suit’, and so on down to the atomic representational elements of the sentence. Following Fodor (2007), I’ll call such privileged parts of discursive representations constituents. The compositional functions of discursive constituents can be understood syntactically as well as semantically: they’re the privileged parts of complex discursive representations that (a) are syntactically ordered in such a way as to yield the whole representation, and (b) possess semantic properties that compose in line with their syntactic ordering to yield the semantic properties of the whole representation. While icons have parts, therefore, they lack canonical decompositions and so lack constituents. Every part of an icon makes both a syntactic and semantic compositional contribution, in line with iconicity, to the whole icon.
The difference between iconic and discursive representations is a difference in representational format, i.e., general type of syntactic structure. Though Green refers to the “representational format” of the representational outputs of structure constancies, it isn’t clear whether he means to refer to the syntactic or semantic properties of those representations. As aforementioned, his primary interest seems to be in the semantics, so I read his use of ‘format’ as looser than mine and will instead use the term to refer solely to types of syntactic structures.
Green may have independent theoretical commitments that lead him to get off the boat at some point in the above discussion, but I’ll assume the syntax–semantics distinction and iconic–discursive distinction, as articulated above, are common ground—though if he has in fact headed for shore this early, it would be helpful for him to say why.
2. The (syntactic) format of hierarchical descriptions
Our primary questions are these: What is the format (i.e., syntactic-structure-type) of the part-centered hierarchical descriptions that are generated by structure constancies? Is it iconic, discursive, or neither? If it’s discursive, is it the same type of format as a propositional thought, or some distinct type of discursive format?
It is hard to see how the kinds of hierarchical descriptions Green describes could possibly be iconic. These representations must specify compositional structures of represented objects, which, Green says, contain
Icons simply aren’t syntactically equipped to specify compositional structures. Consider Figure 2 again. The photograph of Obama is segmentable into the arbitrary shapes A and B precisely because it does not explicitly specify any particular decomposition, nor the “boundaries between connected pairs of parts.”
A proponent of the iconic interpretation could object that icons explicitly specify all possible decompositions, and thus the decomposition into A and B is one among the nearly infinite number of compositional structures represented by Figure 1. In that case, however, if the representations underlying structure constancies are iconic, we should expect structure constancy with respect to every possible decomposition of the perceived scene. As Green notes, this just doesn’t seem to be the case. Green’s phenomenal contrast argument, in fact, relies on some transformations of parts being phenomenologically special in a way that requires one particular compositional structure to be privileged (i.e., explicitly represented) in a way other possible ones aren’t. Icons don’t seem capable of delivering this.
So there is good reason to doubt that the phenomenology of structure constancy is subserved solely by representations in an iconic format. The next question is whether they involve discursive representations, or some other format altogether. There doesn’t seem to be anything that rules out discursive format. The central job description of hierarchical descriptions is to prioritize a particular part decomposition despite the fact that the scene affords a nearly infinite range of possible parts and spatial configurations. Discursive representations are syntactically equipped to privilege certain parts by means of constituents that represent each part. For example, suppose Maria is bizarrely struck with domain-specific amnesia about the configurations of her various body parts; suppose she walks over to a mirror to remedy this unfortunate situation and, looking at her image, thinks MY RIGHT ARM IS ABOVE MY RIGHT LEG. Some of the constituents of that discursive thought are explicit syntactic items (i.e., MY RIGHT ARM and MY RIGHT LEG) that stand for particular parts of Maria. Furthermore, the explicit syntactic constituent ABOVE is used to privilege that particular spatial relation over other possible ones. Thus discursive representations seem to have the requisite syntactic apparatus to instantiate hierarchical descriptions.
While I leave it open that hierarchical descriptions might occur with neither discursive nor iconic format, the considerations in the previous paragraph render that possibility dubious. The appeal to discursive format allows us to explain the privileging of certain parts and spatial relations by appeal to explicit syntactic constituents that stand for those parts and relations. It’s hard (for me, in any case) to imagine how a non-discursive representation could provide the relevant privileging in the explicit way Green argues hierarchical descriptions require. Perhaps Green disagrees, in which case it would be interesting to hear his thoughts.
At this point, we can take it as a working hypothesis that hierarchical descriptions are at least partly discursive. In that case, it might still turn out that the relevant representations are a different sort of discursive representation than the kind that figure in thought. In particular, perhaps they lack propositional discursive format. Not all instances of discursive format are propositional—for example, the linguistic expression ‘black cat’ and the corresponding complex concept BLACK CAT are discursive but don’t feature predication, and so aren’t propositional. Perhaps the representations that instantiate hierarchical descriptions are structured in a similar fashion.
Without more being said in favor of this proposal, it seems doubtful for two reasons. First, it is common ground among representationalists that perceptual representations have accuracy conditions. The concept BLACK CAT is not accurate or inaccurate. The thought THAT IS A BLACK CAT can be accurate or inaccurate because it predicates the property of being a black cat of some particular selected by the demonstrative constituent THAT. The syntactic introduction of predication, however, is what makes the thought syntactically propositional while the concept is not. Thus without propositional format, and hence without predication, it’s hard to see how the relevant discursive representations could have accuracy conditions.2 Second, as Green argues, hierarchical descriptions must encode monadic features of each privileged part and the spatial/mereological relations between parts. Thus the constituents that stand for each part and the ones that stand for the monadic features and spatial/mereological relations must be syntactically connected to each other. Predication is surely capable of this, and in its absence, some other operation must be supplied. Unless one is forthcoming, the propositional interpretation seems superior.
These points emphasize that both the syntax and the semantics of hierarchical descriptions cry out for explanation in terms of predication—the semantics, to explain accuracy conditions, and the syntax, to explain how the relevant constituents are connected in the way they need to be. Inference to the best explanation suggests that we should hypothesize that hierarchical descriptions involve discursive constituents connected via predication; in other words, that they are propositional structures featuring in perception rather than cognition.
The last question to consider here is whether hierarchical descriptions are purely discursive, or whether they arise out of some interaction between discursive structures and earlier generated iconic representations of the perceived scene. Perhaps the discursive structures are overlaid onto icons, in a manner akin to labels and notes scribbled on a treasure map (e.g., ‘This rock is five feet high’, ‘Thompson’s house’, or simply ‘X’).
This notion of “discursive overlay” is, of course, metaphorical. The only way I can think to cash it out would be that the discursive structures inherit or otherwise exploit, in some fashion to be determined, the spatial array supplied by the icon. This seems plausible for other (controversially!) plausible cases of discursive perceptual representations, such as object files or representations of faces (see Quilty-Dunn unpublished). Whether the overlay hypothesis is plausible here, however, depends entirely on the way in which icons represent space. Burge argues that the iconic format that he claims underwrites all perception represents via “an ego-centrically anchored spatial coordinate system” (2014, 494). It also seems intuitively correct that imagistic representations are perspectival, and thus organize space relative to a perceiver’s viewpoint (cf. Peacocke 1992)—this also seems true of photographs, for instance. But as Green argues, structure constancy requires a part-centered spatial framework rather than a view-based one. In that case, discursive hierarchical descriptions cannot inherit the spatial framework of icons, since they require a sui generis spatial framework. Perhaps some of the iconic representations that figure in visual processing involve a part-centered spatial framework, but that proposal (a) needs independent substantiation, and (b) seems implausible given the arguments above that icons don’t seem capable of privileging parts or their decompositions.
One final point, to stem confusion. As mentioned above, Green does talk about hierarchical descriptions as a kind of format, and offers a diagram (figure 9 in his paper) in which the “nodes” correspond to privileged parts and “lines” correspond to mereological and spatial relations. One might read off of this that he is, after all, talking about a sui generis type of syntactic structure that involves nodes and lines and is neither discursive nor iconic. But that would be to mistake the diagram for the mental representation it is used to describe. The point of the diagram is to make it clear that the representation represents parts and their relations in complex ways, which are more easily presented to the reader in a diagram than in words. If he had instead listed all the parts and their relations in words, it would no less accurately model the mental representation. The list and the diagram are simply different ways of conveying the contents of the representation, and as such speak only to its semantics while remaining silent on its syntax.
The conclusion to draw from all of this is that Green appears to have put his finger on a type of representation that has a propositional format and operates independently of iconic representations and yet, if he is right, is proprietarily visual and consciously so. If that’s right, and perception outputs representations with the same sort of syntactic format as thoughts (viz., propositional format), then the perception–cognition border cannot be drawn by appeal to format.
References
Block, N. (2014). Seeing-as in the light of vision science. Philosophy and Phenomenological Research 89(3), 560–572.
Brewer, B. (2011). Perception and Its Objects. Oxford: OUP.
Burge, T. (2010). Origins of Objectivity. Oxford: OUP.
—. (2014). Reply to Rescorla and Peacocke: Perceptual content in light of perceptual constancies and biological constraints. Philosophy and Phenomenological Research 88(2), 485–501.
Carey, S. (2009). The Origin of Concepts. Oxford: OUP.
Fodor, J. (2007). The revenge of the given. In B. McLaughlin and J. Cohen (eds.), Contemporary Debates in Philosophy of Mind (Oxford: Blackwell), 105–116.
Kosslyn, S. M. (1994). Image and Brain. Cambridge, MA: MIT Press.
Martin, M. G. F. (2006). On being alienated. In Perceptual Experience, ed. T. S. Gendler and J. Hawthorne (Oxford: OUP), 354–410.
Peacocke, C. (1992). A Study of Concepts. Cambridge, MA: MIT Press.
Quilty-Dunn, J. (Unpublished). Perception is not (wholly) iconic.
Notes
ICONICITY captures the standard notion of iconic representations as it figures in cognitive science—see, e.g., Kosslyn 1994, 5; Fodor 2007, 108; Carey 2009, 452.
This isn’t to say that predication is required for accuracy conditions. Burge (2010) argues that perception is iconic and has accuracy conditions. How a representational format that lacks the syntactic apparatus for predication is capable of delivering accuracy conditions, however, is a serious lacuna in Burge’s account.
Responses to Hummel and Quilty-Dunn
I am grateful to John Hummel and Jake Quilty-Dunn for their insightful commentaries on my paper. Both have pressed me to think carefully about certain features of my view, and to provide some sorely needed clarifications. Since Hummel and Quilty-Dunn focus on largely distinct issues, I’ll consider the two commentaries separately.
1. Hummel
I have learned a great deal from Hummel’s experimental and theoretical work on structural representation. As such, it is unsurprising that Hummel’s commentary identifies several further lines of support for the claim that vision encodes compositional structure. He also notes an important aspect of my theoretical approach. Let’s start with the latter.
As Hummel observes, my paper places primary emphasis on the problems that the visual system needs to solve (e.g., recognizing objects across different positions and poses, predicting the way a complex object is likely to transform over time), and less emphasis on the neuroscience. However, it should be noted that the view developed in the paper also finds support in neuroscience. In fact, interesting electrophysiological evidence has been produced in favor of the view that neurons in macaque inferotemporal cortex are sensitive to many of the geometrical properties to which classical structural description models appeal, such as axes of symmetry and “nonaccidental” edge properties such as straight vs. curved (see, for example, Vogels et al. 2001; Kayaert et al. 2003, 2005).
Hummel reminds me of earlier work that offers corroborating evidence for the view that visual shape representation is invariant to translations, scaling, and reflection (Biederman & Gerhardstein 1993; Biederman & Cooper 1991). Specifically, visual priming effects due to an initially encountered object were found in these studies to transfer to a stimulus that differed by translation, scaling, or reflection. This would be predicted on the assumption that the very same representation of shape is activated regardless of an object’s absolute position, size, or “handedness.” Moreover, Biederman and Gerhardstein (1993) found that such view-invariant priming benefits seem to depend on the object’s being readily decomposable into parts. This evidence does indeed strengthen my argument against purely viewer-centered models (although exactly how to interpret the evidence is controversial—see Tarr 1995).
I also welcome Hummel’s pointer to Saiki & Hummel (1998). One potential worry with hierarchical approaches to shape description is that as an object becomes more complex, the number of pairwise relations to be encoded grows exponentially. However, this problem can be largely avoided if we assume that the visual system only explicitly represents relations between connected parts. Consistent with this, Saiki and Hummel found that connectedness facilitates the encoding of between-part spatial relations (as evidenced by a reduction in binding errors).
Against my characterization of part decomposition as “involuntary” (p. 16), Hummel cites Thoma et al. (2004), who produced evidence in support of the position that an object is represented hierarchically (in terms of its parts and their spatial relations) only when attention has been cued to the location of the object. Similarly, Hummel’s (2001) influential JIM.3 model of object recognition holds that the generation of hierarchical descriptions occurs only for attended objects, while unattended objects are represented holistically via view-based templates.
I agree (and am further convinced by Thoma et al. 2004) that many aspects of hierarchical description likely require attention. In particular, spatial attention may be needed for the complex binding of multiple properties and relations to an individual part (e.g., Treisman 1988). However, others have contended that part decomposition itself can occur preattentively. Thus, Xu and Singh (2002) argued on the basis of visual search data that objects are decomposed rapidly and in parallel across the visual field. Likewise, Driver and Baylis (1995) have suggested that mirror symmetry is extracted early and preattentively, and that the detection of symmetry depends in turn on decomposing a shape into convex parts. I would be interested to hear Hummel’s take on this evidence.
Finally, Hummel suggests that I may understate the similarity between my position and classic proposals due to Marr and Nishihara (1978) and Biederman (1987). I certainly did not intend to downplay my indebtedness to these theorists. In fact, one of my goals in the paper was to argue that recent empirical evidence vindicates significant components of these views. However, I also want to flag a salient characteristic of the Marr/Biederman approach to which I am not committed. While both of these theorists propose that objects are decomposed into 3-D volumes, the view I develop is neutral about whether the relevant parts are 3-D volumes or instead, say, 2-D surfaces (Leek et al. 2009). This is noteworthy because one frequently offered reason for resisting the view that aspects of visual consciousness are underpinned by Marr’s hierarchical stage (the 3-D model) is that visual experience purportedly does not present us with the hidden “backsides” of objects. Rather, it is held that we only have visual experience of the attributes of visible surfaces (e.g., Prinz 2012: 51-52; 76). However, it is important to recognize that this objection (even if it is persuasive) pertains only to one development of the hierarchical approach. A hierarchical approach is consistent with, but does not require, the representation of volumetric shape properties (cf. Singh & Hoffman 2001).
2. Quilty-Dunn
Quilty-Dunn’s commentary focuses primarily on the implications of my view for the syntactic properties of shape representations. I believe he has gestured toward several further developments of the project. For example, important theoretical progress is likely to result from investigating which kinds of hybrid (partly iconic and partly discursive) representational formats have the resources to represent compositional structure. Quilty-Dunn has also rightly pressed me to clarify some of the central notions put to work in the paper. Here I will primarily focus on two points. First, I’ll clarify what I mean by the notion of “hierarchical format.” Second, I’ll offer some further thoughts on the limitations of a purely iconic approach to shape representation.
However, before doing this, I want to briefly address one part of Quilty-Dunn’s characterization of my account that is potentially misleading. Quilty-Dunn attributes to me the claim that hierarchical descriptions encoding compositional structure are generated by structure constancy (e.g., bottom of p. 3). However, my position is really that hierarchical descriptions encoding compositional structure partially underlie structure constancy. It is because such representations are constructed that the visual system (and, as a result, visual experience) differentiates changes that preserve compositional structure from those that do not. However, with the exception of this detail, Quilty-Dunn summarizes my view correctly.
2.1. A Clarification
Quilty-Dunn writes: “Though Green refers to the “representational format” of the representational outputs of structure constancies, it isn’t clear whether he means to refer to the syntactic or semantic properties of those representations” (p. 3). The representational format Quilty-Dunn has in mind here is the idea of hierarchical description. Let me take this opportunity to clarify my view.
In a few places, I indeed used the notion of “format” to characterize hierarchical description. I now think this was unfortunate. My preferred way to classify hierarchical description is rather as a general type of “scheme” or “code” potentially used by a system of shape representation. A code (e.g., Morse code or the English language) involves (i) a syntax (primitive symbols and rules for combining those symbols into complex representations), (ii) assignments of contents to primitive symbols, and (iii) rules of semantic composition specifying how the contents of complex representations are “built” from the contents of simpler representations. I believe that the view that the visual system constructs hierarchical descriptions of shape places constraints on both the syntax and the semantics of visual shape representation.
For example, it falls out of my view that visual shape representations must have a syntax sufficient for solving the binding problem (Clark 2000; Treisman 1988). Visual shape representations must be able to ascribe multiple properties (e.g., both monadic shape properties and spatial relations) to a single individual (e.g., a single part of an object). As such, visual shape representations must have the resources for appropriately combining representations of properties with representations of individuals (perhaps as linguistic predicates combine with singular terms), and such combination must be semantically significant.
Quilty-Dunn (p. 5) recognizes the need for binding, and suggests that a propositional syntax incorporating predication would be the best way to meet that need. I agree that a full propositional syntax would be up to the task, although it is possible that a more impoverished format would also be sufficient. Burge (2010), for example, suggests that perceptual representations attribute properties to individuals, but do not predicate them. On Burge’s view, the perceptual ascription of a property F to an individual x is accomplished via a representation akin to “That F” (where x is referred to by “that”), rather than via a representation akin to “That is F.” I see no obvious reason why hierarchical descriptions, as I’ve introduced them, must incorporate predication rather than mere attribution. Perhaps Quilty-Dunn has reasons for preferring a predicational approach to representing compositional structure. If so, it would be helpful for him to share them.
As Quilty-Dunn notes, the view defended in the paper has perhaps even more important implications concerning the semantics of the visual system’s shape representation scheme. I argue that such representations must contain symbols that pick out individual parts (the “nodes” in the description). Furthermore, the scheme must incorporate symbols for at least two kinds of relations: mereological (part-whole) relations (“P-edges”) and boundary relations (“B-edges”). Finally, both the intrinsic shapes of parts and the boundary relations between parts must be represented in part-centered coordinates. These are all features of the semantic content of visual shape descriptions.
2.2. Iconic Representations and Their Discontents
I turn now to Quilty-Dunn’s discussion of iconic representation.
The compositional structure of an object involves, inter alia, a privileged decomposition of that object into parts. If a shape representation does not make a particular part decomposition explicit, then it does not prioritize that decomposition relative to the indefinitely many decompositions of the object that are logically possible. In section 4 of my paper, I argued that view-based approaches to shape representation in the object recognition literature (e.g., Ullman & Basri 1991; Edelman 1999) lack the resources to underlie structure constancy because they do not prioritize any particular part structure. Quilty-Dunn contends that a similar difficulty may afflict a purely “iconic” or “depictive” approach to shape representation. I agree (at least on one common construal of iconic representation), and I want to offer some further thoughts on the representational limitations of the iconic approach.
For purposes of concreteness, I will work with the notion of “depictive representation” offered by Kosslyn, Thompson, and Ganis (2006):
We can characterize depictive representations as follows: The syntax: (1) The symbols belong to two form classes: points and empty space. (2) The points can vary in size, intensity, and color. (3) The points can be arranged so tightly as to produce continuous variation, or so sparsely as to be distinct (like the dots in a comic strip). (4) The rules for combining symbols require only that points be placed in a spatial relation to one another. The semantics: (1) The association between a representation and what it stands for is not arbitrary…. That is, (a) each portion of the representation must correspond to a visible portion of the actual object or objects, and (b) the represented “distances” among the portions of the representation must correspond to the distances among the corresponding portions of the actual object. (11-12)
On this approach, icons (like many view-based models) belong to the class of holistic template representations (e.g., Palmer 1978). Quilty-Dunn is correct in claiming that representations of this kind fail to make a specific part decomposition explicit. The symbols contained within the representation—points and empty space—stand for local elements of the representatum (e.g., individual points or small surface patches). But unless supplemented (e.g., by what Quilty-Dunn calls a “discursive overlay”), the representation does not prioritize any particular way of grouping these local elements into larger individuals such as objects or parts. (Of course, we prefer certain segmentations of a picture when we look at it, just as we prefer certain segmentations of visible scenes. But this doesn’t mean that a privileged segmentation falls out of the content of the picture.)
However, the limits of template representation are in fact more pronounced than this, which can be appreciated by reflecting on the geometrical information that a template actually makes explicit. Templates explicitly specify only metric information about an object (e.g., the locations of its surface points and edge elements). Thus, to recover the abstract shape property parallelogram on the basis of a template, one needs to first verify, e.g., that the object represented has two pairs of straight, parallel sides. But holistic templates do not explicitly encode the properties of straightness or parallelism. Such properties need to be computed from the positions of local edge elements. Indeed, if the template is purely local, then it does not even specify the composition of local edge elements into complete “sides.” Thus, abstract shape information (if represented at all) is represented only implicitly (cf. Dretske 1981: ch. 6). Expensive computational work is needed in order to recover it.
The metric properties explicitly encoded by a template are notoriously fragile. They are usually altered by the slightest change of viewpoint on an object. Furthermore, distinct objects from the same category (e.g., cars, dogs, or tables) are almost never associated with precisely the same metric properties. This is a problem for template-based theories because tasks such as basic-level categorization—along, I contend, with several other purely visual tasks—often require the extraction of abstract shape information (e.g., parallelogram or closed figure), none of which is explicitly encoded by a template (see Green 2015 for further discussion).
Quilty-Dunn is skeptical of the idea that iconicity is the diagnostic feature for distinguishing perception from cognition. I share his skepticism, especially if “iconic” is used to denote a purely holistic template-like format.
References
Biederman, I. (1987). Recognition-by-components: A theory of human image understanding.
Psychological Review, 94(2), 115-147.
Biederman, I., & Cooper, E. E. (1991a). Evidence for complete translational and reflectional
invariance in visual object priming. Perception, 20, 585-593.
Biederman, I., & Gerhardstein, P. C. (1993). Recognizing depth-rotated objects: Evidence and
conditions for three-dimensional viewpoint-invariance. Journal of Experimental Psychology: Human Perception and Performance, 19, 1162-1182.
Burge, T. (2010). Origins of Objectivity. Oxford: Oxford University Press.
Camp, E. (2007). Thinking with maps. Philosophical Perspectives, 21, 145-182.
Clark, A. (2000). A Theory of Sentience. Oxford: Oxford University Press.
Dretske, F. I. (1981). Knowledge and the Flow of Information. Cambridge, MA: MIT Press.
Driver, J., & Baylis, G. C. (1995). One-sided edge assignment in vision: 2. Part decomposition,
shape description, and attention to objects. Current Directions in Psychological Science,
4(6), 201-206.
Edelman, S. (1999). Representation and Recognition in Vision. Cambridge, MA: MIT Press.
Feldman, J., & Singh, M. (2006). Bayesian estimation of the shape skeleton. Proceedings of the
National Academy of Sciences, 103(47), 18014-18019.
Green, E. J. (2015). A layered view of shape perception. The British Journal for the Philosophy
of Science. DOI: 10.1093/bjps/axv042
Hummel, J. E. (2001). Complementary solutions to the binding problem in vision: Implications
for shape perception and object recognition. Visual Cognition, 8, 489–517.
Kayaert, G., Biederman, I., & Vogels, R. (2003). Shape tuning in macaque inferior temporal
cortex. The Journal of Neuroscience, 23, 3016-3027.
Kayaert, G., Biederman, I., & Vogels, R. (2005). Representation of regular and irregular shapes
in macaque inferotemporal cortex. Cerebral Cortex, 15, 1308-1321.
Kimia, B.B. (2003). On the role of medial geometry in human vision. Journal of Physiology-
Paris, 97, 155-190.
Kosslyn, S. M., Thompson, W. L., & Ganis, G. (2006). The Case for Mental Imagery. Oxford:
Oxford University Press.
Leek, E. C., Reppa, I., Rodriguez, E., & Arguin, M. (2009). Surface but not volumetric part
structure mediates three-dimensional shape representation: Evidence from part–whole
priming. The Quarterly Journal of Experimental Psychology, 62(4), 814-830.
Marr, D., & Nishihara, H. K. (1978). Representation and recognition of the spatial organization
of three-dimensional shapes. Proceedings of the Royal Society of London, B: Biological Sciences, 200, 269–294.
Palmer, S.E. (1978). Fundamental aspects of cognitive representation. In E. Rosch & B. Lloyd
(eds.), Cognition and Categorization, pp. 261-304. Hillsdale, NJ: Lawrence Erlbaum.
Prinz, J.J. (2012). The Conscious Brain: How Attention Engenders Experience. Oxford: Oxford
University Press.
Saiki, J. & Hummel, J. E. (1998). Connectedness and the integration of parts with relations
in shape perception. Journal of Experimental Psychology: Human Perception and
Performance, 24, 227-251.
Singh, M., & Hoffman, D. D. (2001). Part-based representations of visual shape and
implications for visual cognition. In T.F. Shipley & P.J. Kellman (eds.), From Fragments to Objects: Segmentation and Grouping in Vision. New York, NY: Elsevier Science.
Tarr, M. J. (1995). Rotating objects to recognize them: A case study on the role of viewpoint
dependency in the recognition of three-dimensional objects. Psychonomic Bulletin & Review, 2(1), 55-82.
Thoma, V., Hummel, J. E., & Davidoff, J. (2004). Evidence for holistic representations of
ignored images and analytic representations of attended images. Journal of Experimental Psychology: Human Perception and Performance, 30(2), 257-267.
Treisman, A. (1988). Features and objects: The fourteenth Bartlett Memorial Lecture. The
Quarterly Journal of Experimental Psychology, 40(2), 201-237.
Ullman, S. & Basri, R. (1991). Recognition by linear combinations of models. IEEE
Transactions on Pattern Analysis & Machine Intelligence, 13, 992-1006.
Vogels, R., Biederman, I., Bar, M., & Lorincz, A. (2001). Inferior temporal neurons show greater
sensitivity to nonaccidental than to metric shape differences. Journal of Cognitive Neuroscience, 13, 444-453.
Xu, Y., & Singh, M. (2002). Early computation of part structure: Evidence from visual search.
Perception & Psychophysics, 64(7), 1039-1054.
Hi E.J.,
Thanks for an illuminating and thought-provoking paper!
I want to focus on the issue of part-centered coordinates. You present it as crucial for the experience of structure constancy, and consequently as an important difference between your view and Jackendoff’s.
I was wondering whether it is possible to account for structure constancy without part-centered coordinates. You argue that when a person walks, the location of her joints move relative to viewer-centered coordinates, but structure constancy requires that location be (experienced as) stable, as constant, hence we need part-centered coordinates.
My thought (more of a hunch) is that perhaps we can get structure constancy without representing locations of joints at all (neither viewer-centered nor part-centered). Instead, I think perhaps we can think of the structure of a body as involving no more than joints, lengths of body parts, connections between joints and distance between connected joints. Perhaps a good representation of this is a weighed graph, specifically a tree, with each internal vertex representing a joint, each leaf representing the end of a body part, and each edge representing a connection between these, with weights representing lengths. Perhaps (although this is not crucial) this can be thought of as a sort of abstraction from the medial axis. I assume that the medial axis contains information about locations (and angles), but the tree I suggest does not include any information about location.
Consider now the rabbit case (figure 4 in your paper). Figures 4a and 4b both involve an internal vertex (the head) with two edges (the ears) and two leafs (the tip of each ear). In contrast, figure 4c involves an internal vertex (the head) with one edge (one ear), which ends in a second internal vertex, from which a new edge emerges (the second “ear”), and then one leaf. In this sense, 4a and 4b share structure, while 4a and 4c do not.
Consider also a person walking. It seems that the graph (hence the structure) remains the same throughout her walk, because the connections between joints remain the same, and the lengths of the limbs remain the same and consequently the distances between connected joints remain the same.
I don’t know if this is generalizable to all the cases of structure constancy, or structure inconstancy. Perhaps we need to add additional information to the graph. But at least it appears to put some pressure on the idea that we must appeal to part-centered locations in order to account for structure constancy.
What do you think?
Hi Assaf,
Thanks for this very interesting suggestion! I’ll really need to think it through in detail in order to give a considered evaluation. I agree that it puts some pressure on the idea that we need full-fledged part-centered reference frames in order to explain some of the critical experiential effects. An initial reaction below:
I think that the proposal you mention would indeed be adequate to explain several of the phenomena I gather under the label “structure constancy” (including the rabbit case). However, I’m not convinced that it explains all the cases. For example, I think that changes that leave joint locations intact are experienced very differently from–much less commonly encountered–changes that alter joint locations (e.g., figures 3a-3c). I’m not sure that this would be registered in the proposed account, since the topological configuration of part connections is the same in both cases, and part lengths stay the same as well.
Hummel and Stankiewicz (1996: “Categorical relations in shape perception”) provide some further evidence indicating that perceivers are sensitive to where joints are located in part-centered coordinates. For example, suppose that in figure 1 part A intersects part B above B’s midpoint, while in figure 2 A intersects B below B’s midpoint. This change tends to be much more salient than one where the location of intersection moves by the same amount, but stays above B’s midpoint. This type of effect is (I think) naturally explained on the assumption that the joint’s location is represented relative to the parts themselves, but less obviously explained if its location is represented either in viewer-centered coordinates or not represented at all (as on the current proposal).
There is a lot more to think through here. It might be that certain — more minimal — representational schemes are adequate to explain some of the effects, but richer schemes are needed to explain others.