James R. Beebe and Jake Monaghan (University at Buffalo)
1. Introduction
Epistemic closure principles have figured prominently in contemporary epistemology, particularly in discussions of radical skepticism. The most commonly discussed skeptical challenges in recent decades employ skeptical hypotheses that depict situations that are subjectively indistinguishable from what we take our normal circumstances to be but in which we fail to have knowledge. Where ‘p’ is some proposition we ordinarily take ourselves to know and ‘SK’ is a skeptical hypothesis, these challenges are typically represented as arguments of the following form:
(1.1) If I know that p, then I know that not-SK.
(1.2) I do not know that not-SK.
(1.3) Therefore, I do not know that p.
Premise (1.2) is usually supported by considerations that purport to show that one’s current evidence somehow fails to favor p over SK. Premise (1.1) is usually taken to be an abbreviated substitution instance of the following closure principle for knowledge:
EC1. If S knows that p, and S knows that p entails q, then S knows that q.
Despite its ubiquity, EC1 is widely recognized to be unsatisfactory, since it does not allow that someone may know two things (e.g., that p is true and that p entails q) but may not yet have connected the dots and formed the belief that q is true. Some of the most commonly suggested replacements for EC1 that take this consideration into account include the following:
EC2. If S knows that p, and S knows that p entails q, then S knows (or is in a position to know) that q.
CD1. If S knows that p, competently deduces q from p, and thereby comes to believe q, then S knows that q. (Williamson 2000, 177)
EC2 allows that while knowing that p is true and knowing that p entails q may not always be found in conjunction with knowing that q is true, they will always be found with being in a position to know that q is true. CD1 allows for the same possibility, adding that if one forms the belief that q is true on the basis in question, one’s belief will count as knowledge.
Fred Dretske (1970) and Robert Nozick (1981) (in)famously denied that any closure principle for knowledge was true in their efforts to respond to radical skepticism and to construct satisfactory theories of knowledge. However, it is well known that the tide of epistemological opinion has run strongly against Dretke and Nozick, with most philosophers agreeing that closure is “an extremely intuitive idea” (Hawthorne 2004, 33) and that it captures the seemingly incontrovertible idea that “deduction is a way of extending one’s knowledge” (Williamson 2000, 117). Indeed, many would go further and agree with Richard Feldman’s (1995, 487) oft-quoted claim that the denial of closure was “one of the least plausible ideas to come down the philosophical pike in recent years.”
As John Turri (2015, 3) notes, “closure’s proponents rely heavily on the claim that epistemic closure is a defining feature of ordinary thought and talk about knowledge—that is, of folk epistemology.” When philosophers claim a particular idea is extremely intuitive, it is common for experimental philosophers to wonder how many people actually agree with it and to look for ways to find out.[1] Accordingly, Turri (2015) conducted a series of studies to investigate whether epistemic closure is in fact deeply embedded in folk epistemology. His findings are consistent with individuals endorsing closure for perceptually based beliefs but not for inferential beliefs.
In a between-subjects design, Turri asked participants to read one of two versions of the following vignette:
Car theft. When Mr. Maxwell arrives at work in the morning, he always parks in one of two spots: C8 or D8. Half the time he parks in C8, and half the time he parks in D8. Today Maxwell parked in C8.
It’s lunchtime at work. Maxwell and his assistant are up in the archives room searching for a particular document. Maxwell says, “I might have left the document in my car.” The assistant asks, “Mr. Maxwell, is your car parked in space C8? It’s not unheard of for cars to be stolen.”
The perception version of Car Theft ends with the following words:
Maxwell looks carefully out the window and then responds, “No, my car has not been stolen. It is parked in C8.”
The inference version ends with:
Maxwell thinks carefully for a moment and then responds, “No, my car has not been stolen. It is parked in C8.”
Car Theft is based upon Jonathan Vogel’s (1990) well known discussion of car theft type cases that did more than any other article at the time to convince epistemologists that the rejection of closure was a mistake.
Turri then asked participants to select all of the statements below that are true in the Car Theft vignette[2]:
(2.1) Maxwell knows that his car is parked in C8.
(2.2) Maxwell knows that his car has not been stolen.
(2.3) Maxwell is in the archives room.
(2.4) Maxwell is in his assistant’s office.
Statements (2.3) and (2.4) served as comprehension checks. (2.1) and (2.2) tested for closure because Maxwell is depicted as (i) considering his belief that his car is parked in C8 and the basis upon which he believes this, (ii) reaffirming his belief that the car is indeed parked there, (iii) appreciating that his car’s not being stolen follows from it’s being parked in C8, and (iv) affirming that it has not been stolen on this basis. If participants endorse closure, Turri thinks they should select (2.2) as often as they select (2.1). If, however, they reject closure, they should select (2.2) less often.
In Turri’s fifth study, he gave participants a second vignette that had the same structure as Car Theft:
Computer. When Mrs. Palmer arrives at work in the morning, she always starts her computer and puts it to work analyzing data sets. Today she put her computer to work analyzing demographic data and then walked into her assistant’s office across the hall.
Palmer says to her assistant, “It might take the computer a while to analyze the demographic data.” The assistant asks, “Mrs. Palmer, is your computer analyzing the data? It’s not unheard of for computer hackers to attack the system and crash a computer.”
The perception and inference versions of Computer ended with one of the following statements:
Mrs. Palmer looks carefully through the window into her office and then responds, “No, the hackers have not crashed it. The computer is analyzing data.”
Mrs. Palmer thinks carefully for a moment about the matter and then responds, “No, the hackers have not crashed it. The computer is analyzing data.”
Turri then asked participants to select all of the statements below that are true in the vignette:
(3.1) Palmer knows that the computer is analyzing data.
(3.2) Palmer knows that hackers have not crashed the computer.
(3.3) Palmer is in the archives room.
(3.4) Palmer is in her assistant’s office.
As above, (3.3) and (3.4) served as comprehension checks, while (3.1) and (3.2) tested for participants’ endorsement of epistemic closure for knowledge.
Turri found that participants thought Maxwell both knew that his car was parked in C8 and knew that it had not been stolen in the perception condition. In the inference condition, participants thought that Maxwell knew that his car was parked in C8, but they were significantly less likely to think he knew that it had not been stolen. A similar pattern was found with Computer. Participants were as likely to think Palmer knew that her computer was analyzing data as they were to think that she knew that hackers had not crashed the computer in the perception condition. In the inference condition, however, they were less likely to think that she knew that the hackers had not crashed the computer than they were to think that she knew that the computer was analyzing data. Turri (2015, 14) suggests:
Overall, then, ordinary practice does not endorse an unqualified version of the epistemic closure principle. But it’s arguably consistent with our results that ordinary practice is committed to a source-relative version of the epistemic closure principle. In particular, it’s consistent with our results that a perceptual epistemic closure principle is a defining feature of folk epistemology. This is ironic given that the most famous attempted counterexamples feature perceptually based beliefs (e. g. Dretske’s zebra case).
Although Turri does not ultimately think we can conclude that epistemic closure is not part of folk epistemology on the basis of his results, if the findings he reports prove to be robust, they could challenge the received view in contemporary epistemology that an unrestricted epistemic closure principle is a central and non-negotiable feature of our shared epistemic practices.
There are a few things about Turri’s method of examining closure that we think call for further investigation. One is that Turri believes that both the perception and inference versions of Car Theft depict the protagonist as believing one proposition, seeing that this proposition entails another, and (allegedly) believing the second on the basis of inferring it from the first. However, in the perception version of Car Theft, Maxwell “looks carefully out the window” and sees that his car parked in C8. Like Turri, most philosophers will probably think that he only sees that it is parked in C8 but does not see that it has been stolen. Thus, if he is to believe that his car has not been stolen, it will be necessary for him to draw an inference. However, the metaphysics and epistemology of perception are quite complex, and it is not clear to us that ordinary participants will think that an inference must be made in order for Maxwell to arrive at the belief that his car has not been stolen. In our view, it seems quite likely that many ordinary individuals will think that, in addition to seeing that his car is parked in C8, Maxwell will also see—directly and without the aid of inference (or at least without any inference in addition to those that may already be involved in perception)—that it has not been stolen. It seems quite intuitive to think that the fact that Maxwell is laying eyes on his car directly confirms his belief that his car has not been stolen and unintuitive to think that seeing his car can confirm his belief only if this sensory experience is coupled with the belief that seeing his car entails that it has not been stolen. But if Turri’s participants understand Maxwell as seeing directly that his car has not been stolen, then the perception version of Car Theft will not test individuals’ endorsement of closure at all. Consequently, we may not be able to conclude that ordinary individuals endorse closure for perceptually based beliefs but not for inferential ones. The lesson may simply be that when individuals are presented with clear tests for closure, they fail to endorse it.
The inference version of Car Theft seems better poised to test for folk endorsement of closure because the protagonist is not in a position to directly perceive that the car has not been stolen. This belief must be arrived at by inference. Of course, the protagonist is not in a position to directly verify that the car is parked in C8. Maxwell is described as “think[ing] carefully for a moment” before making an assertion about the car. It is somewhat odd that Turri emphasizes the inferential component in Maxwell’s belief that his car is parked in C8 without acknowledging that his belief is based upon perception and memory as well. Maxwell remembers where he parked his car when he arrived at work in the morning. Furthermore, his memorial belief was ultimately based upon perception themselves. On the basis of what he remembers, together with background beliefs about how likely car thefts are, he makes an inference about whether the car is still parked in C8. Since the ultimate basis of the belief in question is the protagonist’s (perceptually based) memories, it may be that participants reject closure in these cases because of the role of memory or background beliefs play in their justification rather than the role of inference.
A second question we had about Turri’s studies concerned the samples he used in his studies. Proponents of the ‘expertise defense’ (e.g., Sosa 2007, Williamson 2011) complain that when experimental philosophers use undergraduates, Mturk workers, or other adults who have not received formal philosophical training in their studies, the data they obtain are of rather poor quality and consequently fail to tell us anything interesting about how we should view the cases presented in the research materials. The complaint alleges that it is only the reflective intuitions of the suitably trained that would warrant the often surprisingly conclusions some experimental philosophers seek to support. In some concluding remarks, Turri (2015, 15) considers the possibility that epistemic closure is “too subtle or complicated for ordinary people to understand, which is why they exhibit patterns that violate it,” but he notes that “ordinary people are remarkably sensitive to the complex factors that undermine knowledge in Gettier cases (Turri, Buckwalter, & Blouw [2015]), which are at least as subtle and complicated as the epistemic closure principle.”
While we do not endorse the expertise defense as a general response to surprising results in experimental philosophy, we wondered whether non-philosophers who had more formal training in deductive reasoning than the average person might be better able to appreciate the whatever complexities are involved in deductive closure. In particular, we wondered how professional mathematicians—who are like the folk in their general lack of familiarity with debates among philosophers concerning closure and skepticism but unlike the folk in regard to their expertise in deductive reasoning—might respond to Turri’s cases. If non-philosophers fail to indicate that Maxwell knows both that his car is parked in C8 and that his car has not been stolen, it might be due to their lack of sufficient skill in handling entailment relations. In other words, their responses might reflect a performance error rather than the fact that closure is not an important part of folk epistemology. However, if professional mathematicians rejected closure at the same rates as other non-philosophers, we think we would be entitled to greater confidence in believing that this pattern of responses was evidence that an unrestricted closure principle is not in fact part of our core folk epistemic practices.
We performed a series of empirical studies designed to address each of the issues described above. We first replicated Turri’s basic results (Study 1) using Car Theft. In our second study, in order to provide participants with a perceptual case that involved inferring something from a perceptual belief that could not itself be directly verified by perception, we gave participants modified versions of Car Theft and asked them whether Maxwell knows that his car is parked in C8 and whether he knows that it has not been destroyed and replaced by an exact replica. We believe this study presents a more significant test of Turri’s source-relative closure hypothesis than his original Car Theft vignette because Maxwell is no longer able (if he ever was) to have direct perceptual access to the second proposition in question. We found that participants in Study 2 overwhelmingly endorsed closure in both the perception and inference conditions.
In our third study, we compared the knowledge attributions and denials of professional mathematicians with university undergraduates and, in accord with our hypothesis, found that mathematicians endorsed closure for both perceptual and inference beliefs. Also in accord with our expectations, they realized that the reliability of Maxwell’s belief-forming process in the inference condition of Car Theft is much lower than in the perception condition and attributed knowledge at much lower levels in the inference condition. Both findings support our contention that the non-philosophers in Turri’s studies are making a performance error when they attribute and deny knowledge in ways that conflict with the closure principle.
We conclude that an unrestricted closure principle that applies to all beliefs, regardless of source, provides a better model of the patterns of folk knowledge attributions that have been observed than a source-relative closure principle and thus that closure does seem to be a core part of folk epistemology after all.
2. Study 1
In our first study, we attempted to replicate Turri’s (2015) original results. We recruited 120 participants (average age = 35, 34% female, 81% Caucasian, 100% native English speakers) from Amazon’s Mechanical Turk (www.mturk.com) and presented them with either the perception or the inference version of Car Theft.[3] Turri’s first three studies used vignettes that ended with Maxwell stating “No, my car has not been stolen. It is parked in C8.” However, in his fourth study, in an effort to make clear to participants that Maxwell believed that his car had not been stolen because he believed it was parked in C8, Turri has Maxwell respond with “My car is parked in C8, so it has not been stolen.” We used the latter formulation in each of our studies. Turri’s use of this alternative formulation did not lead to a significant difference in participants’ responses, but we thought it was nonetheless preferable to use it in our own.[4]
Participants were asked to indicate the extent to which they agreed or disagreed with each of the following statements, the order of which was counterbalanced:
(2.1) Maxwell knows that his car is parked in C8.
(2.2) Maxwell knows that his car has not been stolen.
Participants were asked to select one of the following seven options as their answer: Completely Disagree, Mostly Disagree, Slightly Disagree, Neither Agree nor Disagree, Slightly Agree, Mostly Agree, and Completely Agree. For purposes of analysis, ‘Completely Disagree’ was coded as ‘1,’ ‘Mostly Disagree’ as 2, and so on. Participant responses are summarized in Table 1.
| Perception | Inference | |
| Positive Knowledge | 6.45*** (1.27) | 6.20*** (1.22) |
| Negative Knowledge | 5.62*** (2.00) | 3.75 (1.99) |
Table 1. Mean levels of agreement (and standard deviations) for each knowledge claim in the perception and inference conditions of Study 1. In all tables, an ‘,’ ‘,’ or ‘’ indicates that the designated mean differs significantly from the neutral midpoint at the .05, .01, or .001 level.
Mean levels of agreement differed significantly from the neutral midpoint in three out of four cases (with very large effect sizes).[5] A two-way mixed ANOVA reveals a significant main effect for source (perception vs. inference) that was medium in size and one for the valence (positive vs. negative) of the knowledge claim in question that was large in size.[6] In other words, participants attributed knowledge in significantly greater numbers in the perception condition than in the inference condition and expressed more agreement with the positive knowledge claim than with the negative knowledge claim. A significant and medium-sized interaction was observed between source and valence.[7] In other words, the lower agreement registered for the negative knowledge claim in the inference condition was the result of more than just the source and valence factors operating independently. There was something special about this particular combination of factors.
There was no significant main effect for gender, and there were no significant interaction effects for gender.[8] Age was positively correlated with negative knowledge attributions in the perception condition (with a medium-sized degree of strength).[9] There were no order effects.[10]
In order to see whether the observed differences in strength of agreement translated into differences in the endorsement of closure, we grouped together all participants who selected the ‘Completely Disagree,’ ‘Mostly Disagree,’ or ‘Slightly Disagree’ into a Disagree (or knowledge-denying) category, all who selected ‘Neither Agree Nor Disagree’ into a Neutral category, and all who selected ‘Slightly Agree,’ ‘Mostly Agree,’ or ‘Completely Agree’ into an Agree (or knowledge-affirming) category. We then looked to see how often participants endorsed or rejected closure. Participants were classified as rejecting closure if they either (i) agreed (with some degree of confidence) with a positive knowledge claim but failed to agree with the negative knowledge claim (either by disagreeing with the latter or remaining neutral about it) or (ii) disagreed (with some degree of confidence) with a negative knowledge claim but failed to disagree with the positive knowledge claim (either by agreeing with it or remaining neutral). Participants’ responses are summarized in Figure 1.
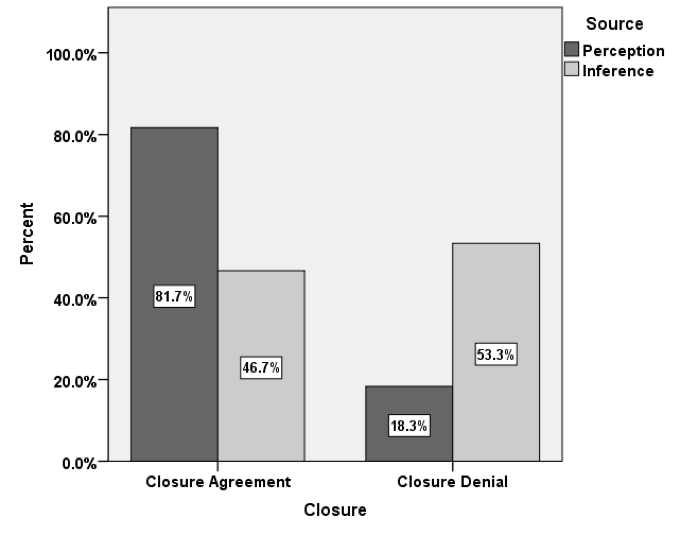
81.7% of participants in the perception condition attributed knowledge in accordance with the closure principle, but 53.3% of participants in the inference condition attributed knowledge in a way that was inconsistent with the principle. These differences are statistically significant, with a medium effect size.[11] There were no gender differences in participants’ rates of the endorsement or rejection of closure in either the perception or the inference conditions.[12] There was a positive correlation between age and the endorsement of closure in the perception condition but not in the inference condition.[13]
Study 1 thus replicates Turri’s initial finding that participants treat positive and negative knowledge claims very differently in the two conditions of Car Theft. Our results are also consistent with the hypothesis suggested (but not endorsed by) Turri that closure applies to perceptually based beliefs but not to inferential ones.
3. Study 2
As we noted above, we harbored doubts about whether Car Theft was a good case to use to test for closure-based knowledge. In the perception version of Car Theft, when Maxwell “looks carefully out the window” and sees that his car parked in C8, we think that many participants will think that Maxwell also sees—directly and without the aid of inference—that it has not been stolen. If this is how participants interpret the vignette, it will fail to serve as a test for closure.
Therefore, in Study 2, we constructed the following variant of Car Theft in which Maxwell comes to believe a proposition on the basis of perception, recognizes that this proposition entails a second proposition that he cannot directly verify by perception, and comes to believe this second proposition on the basis of the perceived entailment:
Replica. Mr. Maxwell lives in a world where wizards like to practice their magical skills by destroying ordinary objects and replacing them with exact replicas. One problem with this is that while the wizards create exact replicas of the objects they destroy, they do not always create replicas of the internal contents of those objects. So, if they destroy your wallet and replace it with a replica, the replacement may not have copies of your money or credit cards in it.
When Mr. Maxwell arrives at work in the morning, he parks his car in his usual parking spot, space C8. At lunchtime, Maxwell and his assistant are up in the archives room searching for a particular document. Maxwell says, “I might have left the document in my car.” The assistant asks, “Mr. Maxwell, is your car still parked in space C8? It’s not unheard of for wizards to destroy ordinary objects and replace them with exact replicas.”
The perception and inference versions of Replica end with one of the following sentences:
Maxwell looks carefully out the window and then responds, “My car is parked in C8, so it has not been destroyed and replaced with an exact replica.”
Maxwell thinks carefully for a moment and then responds, “My car is parked in C8, so it has not been destroyed and replaced with an exact replica.”
Like Car Theft, Replica features Maxwell wondering where a particular document is and thinking that he might have left it in his car. His assistant is again portrayed as raising a certain kind of error possibility—this time that it has been destroyed and replaced by an exact replica rather than that it has been stolen—after which Maxwell confidently affirms that he knows his car’s whereabouts. However, it is much less plausible to think that in the perception condition of Replica Maxwell can directly perceive that the error possibility in question does not obtain. We think this places the perception and inference conditions on a more equal footing in Replica than they are in Car Theft.
After reading one of the versions of Replica, participants were asked the following comprehension question:
Do the wizards always create replicas of the internal contents of the objects they destroy and recreate?
Participants were directed to select either ‘Yes’ or ‘No.’ Those who failed to answer the question correctly had their responses to the positive and negative knowledge claims excluded from the statistical analysis. Participants were then asked to indicate the extent to which they agreed or disagreed with each of the following claims, the order of which was counterbalanced:
(2.1) Maxwell knows that his car is parked in C8.
(2.5) Maxwell knows that his car has not been destroyed and replaced with an exact replica.
Participants were given the same answer choices as before.
120 participants (average age = 37, 45% female, 71% Caucasian, 95% native English speakers) were recruited from Amazon’s Mechanical Turk, 11 of whom failed to answer the comprehension question correctly. The responses of the remaining participants to the positive and negative knowledge claims are summarized in Table 3.
| Perception | Inference | |
| Positive Knowledge | 4.28 (2.27) | 4.58 (2.16) |
| Negative Knowledge | 4.08 (2.48) | 4.50 (2.30) |
Table 2. Mean levels of agreement (and standard deviations) for each knowledge claim in the perception and inference conditions of Study 2.
In sharp contrast to the results of Studies 1 and 2 and Turri’s original studies, none of the mean levels of agreement differed significantly from the neutral midpoint[14], and neither the source of belief nor the valence of the knowledge claim had any appreciable impact upon participants’ responses.[15] In other words, on the whole participants were not inclined to think that Maxwell knows either that his car is parked in C8 or that it has not been destroyed and replaced with an exact replica. They were also not significantly inclined to deny these knowledge claims. There were no gender effects[16] and no order effects[17], and age did not correlate with agreement to positive or negative knowledge claims in either the perception or inference conditions.[18]
It is crucial to note that the positive knowledge claim used in Study 3 is identical to the positive knowledge claims used in previous studies, and Maxwell’s evidence for this claim is identical as well. The only things that have changed are the relevant alternative that is incompatible with Maxwell knowing where his car is parked, Maxwell’s inability in the perception condition to have direct perceptual access to the falsity of this alternative, and possibly how difficult it is for Maxwell to rule out this alternative with his present evidence.
Although further investigation is required, we believe that the results of our third study support our contention that the perception condition of Turri’s original Car Theft vignette does not serve as a good test case for closure-based knowledge. When Maxwell can look out the window and see that his car is parked in C8, participants may not think there is a need for him to infer that it has not been stolen. They may view him as being able to see this directly. The probability that Maxwell’s car has not been stolen, given that he is looking directly at what appears to be his car, seems quite high. In Replica, however, the probability that his car has not been destroyed and replaced by an exact replica, given that he is looking directly at what appears to be his car, does not seem nearly as high. Participants seemed to appreciate this fact, inasmuch as they were significantly less inclined to think Maxwell knows that the relevant alternative in Study 3 does not obtain, even in the perception condition. And this in turn led participants to be less inclined to think Maxwell knows that his car is parked in C8.
When we looked at how often participants endorsed or rejected closure in Study 2, we failed to observe the patterns that the source-relative closure hypothesis would lead us to expect (cf. Figure 2).
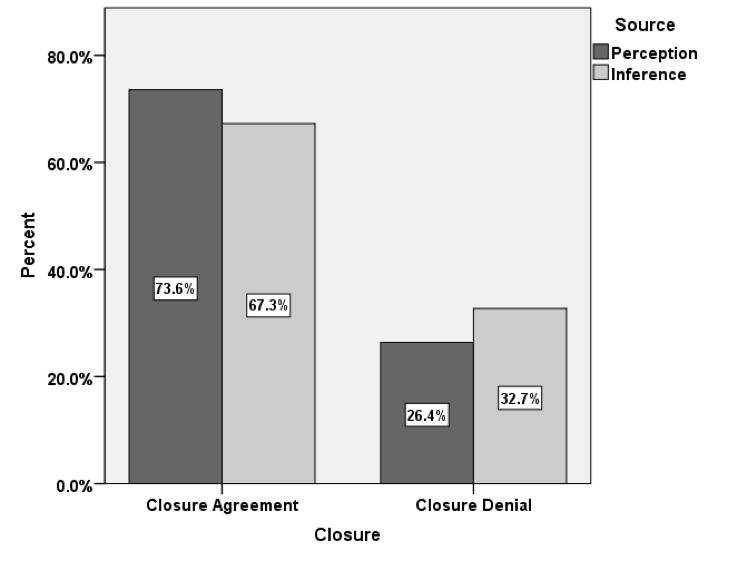
Roughly three-fourths of participants in the perception condition and two-thirds in the inference condition attribute knowledge in ways that were consistent with closure. The differences between these distributions failed to be significant.[19] There were no gender differences in participants’ acceptance or rejection of closure and no correlations between age and closure.[20]
Turri (2015) suggested that folk epistemology might include within it a source-relative closure principle that applies to perceptually based beliefs but not to inferential beliefs. He noted that this possibility accords with data showing that individuals attribute knowledge in accordance with closure far more often in the perception condition of Car Theft than in the inference condition. In the present study, however, we find no difference in participants’ knowledge attributions in the perception and inference conditions and no difference in the frequency of closure agreement and closure denial in the perception and inference conditions. When we compared the perception data from Study 1 to the perception data from Study 2 and the inference data from Study 1 to the inference data from Study 2 using two chi squared tests of independence, we found that the frequency of closure denial in Study 2 was not significantly different than in Study 1 in the perception condition but was significantly lower in the inference condition, with a small effect size.[21]
The source-relative closure hypothesis should not be misinterpreted as the thesis that individuals never attribute or deny knowledge in ways that are consistent with the closure principle. Because the closure principle is supposed to apply to all pairs of propositions, the rejection of closure simply means that it does not always hold. Nevertheless, it does not seem that the hypothesis is able to explain why most of our participants attributed knowledge in accordance with the closure principle in both the perception and inference conditions in Study 3. The explanation cannot be that it is rather difficult for Maxwell to rule out the relevant alternative in the inference condition with his present evidence.
4. Study 3
In Section 1, we noted that Turri (2015, 15) considers the possibility that epistemic closure is “too subtle or complicated for ordinary people to understand, which is why they exhibit patterns that violate it.” Although Turri does not think this possibility underlies the actual pattern of results he obtained, we suggested that non-philosophers might failed to attribute knowledge in accordance with the closure principle either because they reject closure or because they lacked sufficient skill in handling whatever subtlely or complexity is involved in reasoning in accordance with the closure principle. We hypothesized that if closure were a central feature of folk epistemology, non-philosophers who had formal training in deductive reasoning would thus attribute knowledge in ways that were more consistent with the closure principle than the average undergraduate or Mturk worker who lacked the relevant training.
Consequently, for Study 3, we recruited two groups of participants. One group was composed of 254 undergraduates from a large public institution in the northeastern United States (average age = 20, 63% female, 61% Caucasian, 81% native English speakers). The second set of participants were 208 professional mathematicians from large and regional public institutions across the United States (average age = 48, 14% female, 73% Caucasian, 73% native English speakers). The mathematicians were recruited via personal email invitation. Their emails were obtained from the webpages of their respective departments. 58% of them claimed some area of discrete mathematics as their primary area of specialization, with 14% in analysis and 18% in applied mathematics. In addition to being trained in formal methods of reasoning, we also presumed these mathematicians would be more intelligent than the average person and thus would might commit fewer performance errors in their application of their concept of knowledge than ordinary participants.
Each participant in Study 3 was given two vignettes to read and evaluate. Participants received either the perception version of Car Theft and the inference version of Computer case or the inference version of Car Theft and the perception version of Computer. The order with which participants responded to these vignettes was counterbalanced. Each participant who received a Car Theft vignette was then asked to indicate the extent to which they agreed or disagreed with (2.1) and (2.2), and each participant who received some version of Computer was asked whether they agreed or disagreed with (3.2) and (3.3). The order of these statements was also counterbalanced. Participant responses were recorded in the same fashion as in the studies above and are summarized in Table 3.
| Car Theft (Perception) | Car Theft (Inference) | Computer (Perception) | Computer (Inference) | ||
| Undergraduates | Positive Knowledge |
6.16*** (1.32) |
5.70*** (1.83) |
5.74*** (1.42) |
5.26*** (1.86) |
| Negative Knowledge |
5.19*** (1.89) |
3.52* (2.12) |
4.90*** (1.93) |
3.92 (1.99) |
|
| Mathematicians | Positive Knowledge |
6.14*** (1.34) |
4.13 (2.47) |
5.14*** (2.02) |
3.69 (2.37) |
| Negative Knowledge |
5.44*** (1.89) |
2.46*** (1.97) |
4.65** (2.14) |
2.93*** (2.09) |
Table 3. Mean levels of agreement (and standard deviations) for each participant group and each knowledge claim in the perception and inference conditions of Study 3.
The mean levels of agreement differed from the neutral midpoint in thirteen of the sixteen cases.[22] A four-way mixed ANOVA revealed a significant main effect for each of the following independent variables: group (undergraduates vs. mathematicians), vignette (Car Theft vs. Computer), source (perception vs. inference), and valence (positive vs. negative).[23] In other words, (i) there were significant differences in how undergraduates and mathematicians responded to the research materials, with mathematicians reporting lower levels of agreement with all knowledge claims, (ii) participants were less inclined to attribute knowledge in Computer than in Car Theft, (iii) participants attributed knowledge less often in the inference conditions than in the perception conditions, and (iv) participants expressed lower levels of agreement with negative knowledge claims than with positive knowledge claims.
The following two-way interactions were also significant: group x source, group x valence, vignette x source, vignette x valence, and valence x source.[24] In other words, the two groups of participants responded differently to information regarding the source of the protagonist’s belief and the valence of each knowledge claim. Furthermore, how participants were affected by the valence of the knowledge claims determined in part by which vignette they read and the source of the protagonist’s belief.
The most important finding at this stage of analysis concerns the very different ways that undergraduates and mathematicians responded in the two inference conditions. In the inference versions of Car Theft and Computer, undergraduates strongly agreed with the positive knowledge claims that Maxwell knows that his car is parked in C8 and that Palmer knows that the computer is analyzing data. By contrast, mathematicians’ responses to these knowledge claims did not differ from chance. In response to the negative knowledge claims that Maxwell knows his car has not been stolen and that Palmer knows that hackers have not crashed the computer, undergraduates’ responses did not differ from chance, while the responses of mathematicians fell significantly below chance. In short, mathematicians were much more skeptical about the protagonist’s ability to obtain knowledge of the propositions in question simply by thinking carefully about the matter for a moment. We think this is the correct perspective to take on the inference-based knowledge claims, given the lower reliability of thinking carefully for a moment, as compared to looking carefully out the window directly at the objects in question.
As in Studies 1 and 2, we recoded those participants who expressed some level of agreement (‘Completely Agree,’ ‘Mostly Disagree,’ and ‘Slightly Disagree’) into an Agree category, those who expressed some level of disagreement into a Disagree category, and those who neither agreed nor disagreed into a neutral category. We then looked at the relative frequency with which undergraduates and mathematicians endorsed or rejected closure in their assessments of the positive and negative knowledge claims. As before, anyone who agreed with both the positive and negative knowledge claims or who disagreed with both was categorized as endorsing closure. Participants were classified as rejecting closure if they either agreed with a positive knowledge claim but failed to agree with the negative knowledge claim (either by disagreeing or remaining neutral) or if they disagreed with a negative knowledge claim but failed to disagree with the positive knowledge claim (either by agreeing or remaining neutral). Participants who did none of these things were placed into an ‘Other’ category. The results of this analysis are summarized in Figures 3 and 4.
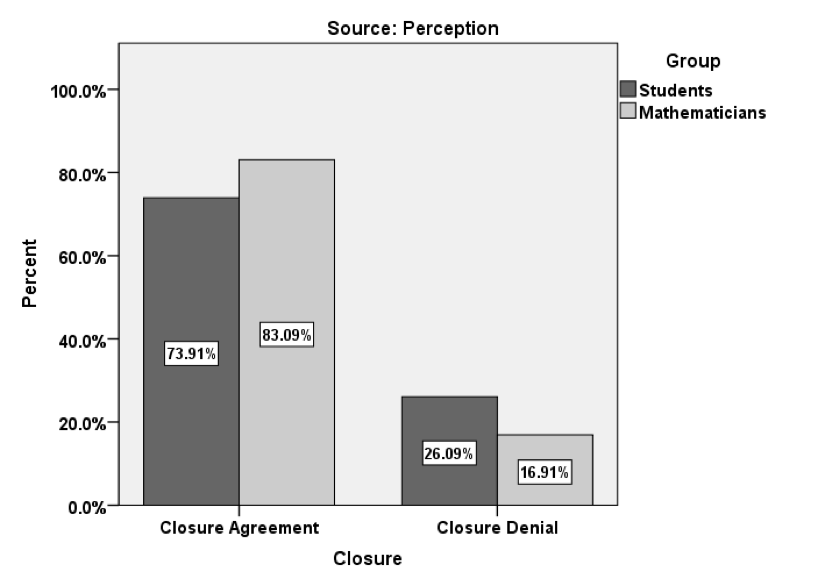
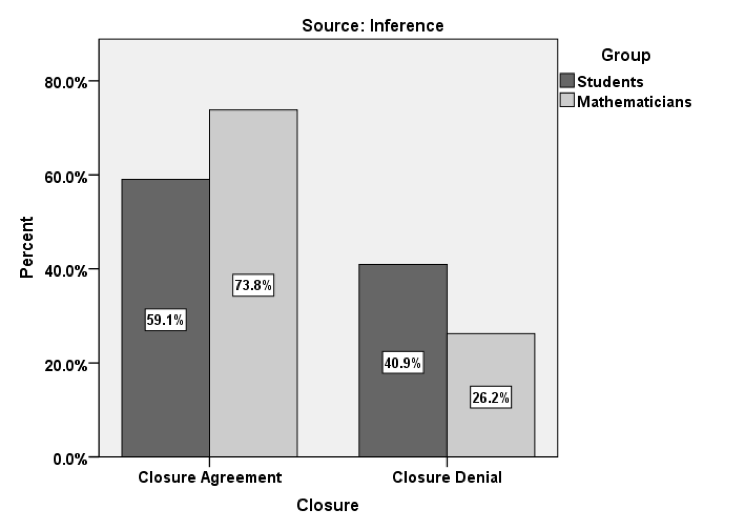
Mathematicians were much less likely to reject closure in either the perception or inference conditions. Two chi-squared tests of independence conducted separately on the perception and inference data revealed that the differences between undergraduates and mathematicians in both conditions were significant.[25] Mathematicians did reject closure at higher rates in the inference conditions than in the perception conditions, but note that mathematicians’ rejection of closure in the inference conditions did not appreciably exceed undergraduates’ rejection of closure in the perception conditions.
According to the source-relative closure hypothesis, our shared epistemic practices include a closure principle that applies to perceptually based beliefs but not to inferential beliefs. The mathematicians that participated in Study 5 are folk-like in the fact that they are not actively involved in philosophical debates about closure and related matters (e.g., skepticism), but they are unlike ordinary folk in regard to their expertise concerning deductive reasoning. If the source-relative hypothesis were true, there should not be any reason why their formal training should lead them to depart from the folk epistemological dictum that closure only applies to perceptually based beliefs. But they do not roundly reject the application of closure to inferential beliefs.
5. Discussion
Violations of closure were observed in the inference conditions of Turri’s original studies and Study 1 but were not found in Study 2. Violations of closure among mathematicians were significantly less common than among undergraduates or Mturk workers. We believe that violations of closure represent performance errors on the part of participants who are insufficiently skilled in appreciating deductive logical relations between propositions. We believe that when participants declined to attribute negative knowledge to Maxwell in the inference conditions, they were giving the correct answer but that they should have refused to attribute positive knowledge to him as well. We believe that an unrestricted closure principle—one that applies to all beliefs, regardless of their source—provides a better model of folk patterns of knowledge attribution than the source-relative closure principle suggested by Turri (2015).
References
Dretske, F. 1970. “Epistemic Operators.” Journal of Philosophy 67: 1007-23.
Feldman, R. 1995. “In Defence of Closure.” Philosophical Quarterly 45: 487-494.
Hawthorne, J. 2004. Knowledge and Lotteries. Oxford: Oxford University Press.
Nozick, R. 1981. Philosophical Explanations. Cambridge, MA: Harvard University Press.
Sosa, E. 2007. “Experimental Philosophy and Philosophical Intuition.” Philosophical Studies 132: 99-107.
Turri, J. 2015. “An Open and Shut Case: Epistemic Closure in the Manifest Image.” Philosophers’ Imprint 15: 1-18.
Turri, J., W. Buckwalter, and P. Blouw. 2015. “Knowledge and Luck.” Psychonomic Bulletin and Review 22: 378-390.
Vogel, J. 1990. “Are There Counterexamples to the Closure Principle?” In M. D. Roth & G. Ross (eds.), Doubting: Contemporary Perspectives on Skepticism. Dordrecht: Reidel, pp. 13-27.
Williamson, T. 2000. Knowledge and Its Limits. Oxford: Oxford University Press.
Williamson, T. 2011. “Philosophical Expertise and the Burden of Proof.” Metaphilosophy 42: 215-229.
Notes
[1] Turri (2015, 3) writes, “This rather lavish gloss on ordinary practice and what ‘we’ find intuitively compelling or conversationally ‘abominable’ has thus far gone unchallenged, even by closure’s opponents (Nozick 1981, 205-6; Dretske 2013, 32). Thus it is widely assumed that closure’s opponents bear the revisionist’s burden of either explaining away contrary intuitions or showing that their view delivers otherwise unattainable benefits.”
[2] In Turri’s first study, he presented participants with both (2.3) and (2.4) and either (2.1) or (2.2). In his second study, Turri presented each participant with all four statements. In a third study, he presented participants with conjunctions that combined (2.1) and (2.2), the negation of only one of these statements, or the negations of both. The overall pattern of data obtained in these different ways remained roughly the same.
[3] Mturk workers in all studies were required to have at least a 98% approval rating on at least 5,000 tasks and were paid $.35 for their participation. Workers were prevented from participating in more than one study in this paper.
[4] In a fifth study that used Computer, Turri has Palmer state “No, the hackers have not crashed it. The computer is analyzing data.” In Study XX below, where we use Computer, we changed this to “The computer is analyzing the data, so hackers have not crashed it.”
[5] Perception/positive knowledge: t(59) = 14.97, p < .001, r = .89. Perception/negative knowledge: t(59) = 6.26, p < .001, r = .63. Inference/positive knowledge: t(59) = 13.99, p < .001, r = .88. Inference/negative knowledge: t(59) = -.97, p > .05.
[6] Source: F(1, 118) = 19.57, p < .001, r = .38. Valence: F(1, 118) = 77.38, p < .001, r = .63.
[7] F(1, 118) = 18.76, p < .001, r = .37.
[8] Main effect: F(1, 115) = .01, p > .05. Gender x source: F(1, 115) = 1.05, p > .05. Gender x valence: F(1, 115) = .46, p > .05.
[10] A three-way (source * valence * order) mixed ANOVA showed no main effect for order, F(1, 116) = .53, p > .05.
[11] χ2 (1, N = 120) = 15.98, p < .001, Cramér’s V = .37.
[12] Perception: χ2 (1, N = 60) = .89, p > .05. Inference: χ2 (1, N = 59) = .32, p > .05.
[13] Perception: r = .29, p < .05. Inference: r = .10, p > .05.
[14] Perception/positive knowledge: t(52) = .91, p > .05. Perception/negative knowledge: t(52) = .22, p > .05. Inference/positive knowledge: t(54) = 2.00, p = .051. Inference/negative knowledge: t(55) = 1.62, p > .05.
[15] Source: F(1, 106) = 1.46, p > .05. Valence: F(1, 106) = .19, p > .05. Source * valence: F(1, 106) = .04, p > .05.
[16] A three-way (source * valence * gender) mixed ANOVA showed no main effect for gender, F(1, 104) = 1.62, p > .05.
[17] A three-way (source * valence * order) mixed ANOVA showed no main effect for order, F(1, 104) = .72, p > .05.
[18] Perception/positive knowledge: r = .15, p > .05. Perception/negative knowledge: r = -.06, p > .05. Inference/positive knowledge: r = -.04, p > .05. Inference/negative knowledge: r = .14, p > .05.
[19] χ2 (1, N = 108) = .52, p > .05.
[20] Gender/perception: χ2 (1, N = 53) = .68, p > .05. Gender/inference: χ2 (1, N = 55) = .08, p > .05. Age/perception: r = -.08, p > .05. Age/inference: r = .16, p > .05.
[21] Perception: χ2 (1, N = 113) = 1.07, p > .05. Inference: χ2 (1, N = 115) = 4.96, p < .05, Cramér’s V = .21.
[22] Undergraduates/Car Theft/perception/positive knowledge: t(127) = 18.54, p < .001, r = .85 Undergraduates/Car Theft/perception/negative knowledge: t(95) = 7.45, p < .001, r = .61. Undergraduates/Car Theft/inference/positive knowledge: t(125) = 10.42, p < .001, r = .68. Undergraduates/Car Theft/inference/negative knowledge: t(125) = -2.53, p < .05, r = .22. Undergraduates/Computer/perception/positive knowledge: t(125) = 13.73, p < .001, r = .78. Undergraduates/Computer/perception/negative knowledge: t(124) = 5.19, p < .001, r = .42. Undergraduates/Computer/inference/positive knowledge: t(127) = 7.66, p < .001, r = .56. Undergraduates/Computer/inference/negative knowledge: t(127) = -.45, p > .05. Mathematicians/Car Theft/perception/positive knowledge: t(96) = 15.78, p < .001, r = .85. Mathematicians/Car Theft/perception/negative knowledge: t(95) = 7.45, p < .001, r = .61. Mathematicians/Car Theft/inference/positive knowledge: t(109) = .54, p > .05. Mathematicians/Car Theft/inference/negative knowledge: t(109) = -8.20, p < .001, r = .62. Mathematicians/Computer/perception/positive knowledge: t(110) = 5.96, p < .001, r = .49. Mathematicians/Computer/perception/negative knowledge: t(110) = 3.20, p < .01, r = .29. Mathematicians/Computer/inference/positive knowledge: t(96) = -1.29, p > .05. Mathematicians/Computer/inference/negative knowledge: t(95) = -5.02, p < .001, r = .46.
[23] Group: F(1, 912) = 44.66, p < .001, r = .22. Vignette: F(1, 912) = 8.31, p < .01, r = .10. Source: F(1, 912) = 180.97, p < .001, r = .41. Valence: F(1, 912) = 280.34, p < .001, r = .48.
[24] Group * source: F(1, 912) = 27.40, p < .001, r = .17. Group * valence: F(1, 912) = 10.70, p < .01, r = .11. Vignette * source: F(1, 912) = 7.79, p < .01, r = .09. Vignette * valence: F(1, 912) = 15.26, p < .001, r = .13. Valence * source: F(1, 912) = 29.25, p < .001, r = .18. There was also a significant three-way interaction between vignette, source, and valence: F(1, 912) = 7.27, p < .01, r = .09.
[25] Perception: χ2 (2, N = 460) = 9.10, p < .05, Cramér’s V = .14. Inference: χ2 (2, N = 460) = 11.20, p < .01, Cramér’s V = .16.

Contemporary Anglophone philosophers often claim that knowledge is “closed under known entailment.” This is glossed as the epistemic closure principle. What does this principle mean? As a first approximation, it means that if you know that some proposition (P) is true, and you also know that if P is true then some other proposition (Q) is also true, then you also know that Q is true. Or, at least, you are in a position to know that Q is true by simply inferring Q from the relevant background knowledge. The epistemic closure principle plays a large role in contemporary epistemological debates, but it is rarely if ever supported by positive argumentation. Instead, it is claimed that the principle is extremely intuitive and an essential part of our ordinary knowledge concept. If a theory of knowledge implies that knowledge is not closed under known entailment, then it is rejected as unacceptably counterintuitive and revisionary (e.g. Hawthorne 2004).
If the epistemic closure principle is an essential part of our ordinary knowledge concept, then we would expect to find evidence of that fact in ordinary knowledge judgments. More specifically, we would expect to find evidence of at least two things. First, we would expect extensional adequacy: people’s knowledge judgments will not contradict what the epistemic closure principle implies about specific cases. Second, we would expect intensional adequacy: people represent the knowledge relation as closed under known entailment. The principle is, one might say, cognitively real. If we found evidence of both those things, then it would be reasonable to infer that a commitment to the epistemic closure principle explains patterns in ordinary knowledge judgments. And this is exactly what philosophical proponents of the epistemic closure principle have claimed.
Researchers recently began testing for extensional adequacy (Turri 2015a, Turri 2015b). A series of recent studies revealed a central tendency for people’s judgments to contradict what the epistemic closure principle implies. This “closure-violating” pattern occurred when participants judged combinations of inferential beliefs with certain qualities. For example, participants read a story about an office worker who is asked where his car is parked and reminded that car thefts sometimes occur. The worker “thinks carefully” for a moment and answers that his car is parked in the lot (call this the premise belief), so it has not been stolen (call this the conclusion belief). The premise belief has positive content: it states that something is true. The conclusion belief has negative content: it states that something is not true. Participants tended to count the premise belief as knowledge, but they tended to not count the conclusion belief as knowledge. The same basic closure-violating pattern occurred across different narrative contexts and questioning procedures. We would not expect this if people were committed to the epistemic closure principle. By contrast, in closely matched scenarios where instead of thinking carefully, the worker “looks carefully,” participants counted both the premise belief and the conclusion belief as knowledge.
In their admirable paper, “Epistemic Closure in Folk Epistemology,” James Beebe and Jake Monaghan advance our understanding of this topic in at least two ways. First, they note that prior research did not test the version of the closure principle that focuses on being in a position to know. This is an important limitation. So Beebe and Monaghan retested some cases but, crucially, probed participants specifically about whether the agent was in a position to know the relevant claim. They observed a closure-violating pattern similar to the one observed in previous research, thereby providing evidence that the logically weaker version of the closure principle is not part of our ordinary knowledge concept either.
Second, they raise the interesting possibility that people might represent the knowledge relation as closed under known entailment despite producing closure-violating combinations of judgments. Otherwise put, Beebe and Monaghan take seriously the possibility that the epistemic closure principle might be intensionally adequate but extensionally inadequate. This could happen because of performance error. For any number of reasons, it might be more difficult to evaluate inferential beliefs than perceptual beliefs, which in turn could produce more closure-violating patterns for inference. So Beebe and Monaghan tested a population with extensive experience evaluating inferences: professional mathematicians. I commend the authors for this very creative idea!
The results suggest that professional mathematicians attribute knowledge differently from laypeople. Nevertheless, despite their extensive education, training, and experience in evaluating inferences, professional mathematicians were still significantly more likely to count inferential beliefs as knowledge when the content was positive rather than negative. This finding suggests that previously observed closure-violating patterns were not due to performance error. More importantly, and independently of any philosophical debates surrounding closure, the finding constitutes surprising evidence that there is something fundamentally different in the way humans evaluate positive versus negative inferential beliefs. It would be not only fitting but also very exciting if this finding inspired further work on the issue, including research examining the effect’s robustness cross-culturally and developmentally.
My final comment on Beebe and Monaghan’s stimulating paper pertains to the second study. This study did not detect a closure-violating pattern in knowledge judgments about a different scenario involving wizards. Beebe and Monaghan attribute this to the fact that the scenario made it easier for participants to appreciate important features of the case. However, an alternative interpretation is suggested by the fact that there was no effect of condition and, overall, mean knowledge attribution hovered just above midpoint. The alternative is that the wizardry scenario was conspicuously extravagant and unrealistic, which incited ambivalence, thereby masking the underlying effect and leaving just a weak agreement bias in all the conditions.
In closing, I thank Beebe and Monaghan for their valuable contribution.
Acknowledgments — For helpful comments and feedback, I thank Wesley Buckwalter, Blake Freier, Ashley Keefner, Dylon McChesney, and Angelo Turri. This research was supported by the Social Sciences and Humanities Research Council of Canada, the Ontario Ministry of Economic Development and Innovation, and the Canada Research Chairs program.
References
Hawthorne, J. (2004). Knowledge and lotteries. Oxford: Oxford University Press.
Turri, J. (2015a). An open and shut case: epistemic closure in the manifest image. Philosophers’ Imprint, 15(2), 1–18.
Turri, J. (2015b). Skeptical appeal: the source-content bias. Cognitive Science, 39(2), 307–324.
John, thanks for your comments. You suggest that our wizarding scenario is “conspicuously extravagant and unrealistic,” and Wesley refers to “the fanciful nature of the stimuli.” Both of you then express skepticism about the sort of conclusions that can be drawn from such a scenario. That’s a fair point. Thanks for raising it. The fanciful nature of the thought experiment does seem to reduce the confidence we can have any conclusions we wish to draw from it.
John takes the fact that “professional mathematicians were still significantly more likely to count inferential beliefs as knowledge when the content was positive rather than negative” to suggest that “previously observed closure-violating patterns were not due to performance error.” It certainly seems to count as evidence of the robustness of the source-content bias that John has identified. But it’s not clear to me that we can draw the conclusion about closure and performance errors that John wishes to draw. Of course, there isn’t yet enough evidence to draw the conclusion I would like to draw either, viz., that the source-content bias is so strong that it can lead trained deductive reasoners to violate a core principle of folk epistemology (i.e., closure).
Hi, James. I think you’re right that it would probably be too strong to flat-out draw that conclusion based on the finding regarding professional mathematicians. But I do think it makes that conclusion more likely, since the population in question has so much training in evaluating inferences. That’s no accident, of course, because it’s precisely why you studied that population to begin with!
For the uninitiated, Turri’s source-content bias is the finding that people are less inclined to attribute knowledge when the source of belief is inference rather than perception and the content of the belief is negative (e.g., not-p) rather than positive (e.g., p). These two factors interact to have a larger effect together than the sum of their individual or independent effects.
John, I thought at one point you were thinking that closure was a core part of folk epistemology after all and that people were making some kind of mistake in not following closure. I would be interested to hear what your view on this is. Thanks
Hey, James. When I first started researching the topic, I was basically agnostic on whether it was a core part of folk epistemology. If I had to guess, I would have guessed that it wasn’t, perhaps partly due to inertia from NHST 🙂 … but mainly because I had a hard time imagining why our knowledge concept would have incorporated a principle of that sort to begin with, and the fact that no other mental-state concept seems to incorporate such a principle. Nevertheless, I took seriously the concern that people were making a mistake of some sort, which is what motivated testing different narrative contexts and procedures.
Does the psychology of knowledge attribution support the principle of epistemic closure? According to prior experimental findings (“An Open and Shut Case: Epistemic Closure in the Manifest Image”), it depends. It may for certain kinds of beliefs, involving perception, but not others, involving inference. The present research team (“Epistemic Closure in Folk Epistemology”) denies this. They argue closure is supported for all beliefs, perceptual and inferential. To undermine the prior experimental findings, they conduct new experiments motivated by two claims:
Claim 1: Prior research failed to demonstrate adherence to closure for perceptual belief.
Since prior research supported perceptual closure, this objection could potentially undermine the present team’s own conclusions. Nevertheless, it can be useful to test the limits of established effects in new conditions. To do this, the researchers tested judgments in new conditions involving magical worlds where wizards create and destroy objects. The researchers found that participants attribute knowledge to characters in these worlds at chance rates, or at about the midpoint of their scale, for each item tested. This rendered null results for the variables manipulated.
It is unclear what can be inferred about the reliability of past findings from this result. Stemming from the fanciful nature of the stimuli, there could be many reasons why attribution in magical worlds occurred at chance rates incidental to closure. In any event, knowledge was not initially attributed in these cases, so an optimal test of closure cannot be preformed. How can one evaluate what follows from a knowledge attribution in folk psychology if no attribution is present in the first place?
Claim 2: Violations of closure observed in prior research are performance errors resulting from insufficient logical ability.
The authors demonstrate that mathematicians attribute knowledge differently than the general population does. However it is unclear how this undermines prior data, for three reasons. First, mathematicians show a similar albeit smaller closure effect as laypeople do. Both groups reject closure at higher rates for inferential than perceptual beliefs. Second, no evidence is given that differences are the result of logical ability. Differences could arise for many reasons and this must be demonstrated before errors are posited. Third, whether a practice is an error is a separate question from whether that practice occurs. The discovery of differences between groups is a valuable contribution in its own right and may have implications for philosophical arguments about closure. But this does not undermine prior observations of ordinary behavior. Lastly, this paper raises three general questions worthy of discussion. The first involves realism in experimental tasks. To what degree should the study of ordinary practice limit experimental materials to the realities of ordinary life? The second involves population. Does sampling from specific groups like professional academics facilitate better or worse measurement of human psychology than randomly sampling the general public does? The third involves undermining data. When does a different result in a new circumstance erase a prior discovery in another circumstance?
Wesley, you characterize us as attempting to “undermine the prior experimental findings” and “undermine prior data.” But that’s not how we understand ourselves. We thought of ourselves as trying to explore one kind of explanation of prior data–one based upon a performance error hypothesis. You ask, “When does a different result in a new circumstance erase a prior discovery in another circumstance?” We never claimed that we were erasing or trying to erase a prior discovery. Instead, we were trying to explore one explanation of it. If people don’t attribute knowledge in accordance with the closure principle, wouldn’t it be good to know why they are failing to do so?
I appreciate you guys pressing us on the conclusion we would like to draw from our mathematician data. Because mathematicians violate closure a good bit less often than ordinary folk (only 26% in the inference condition and 17% in the perception condition), Jake and I wanted to conclude that they largely attribute knowledge in accordance with closure. It’s good for you guys to emphasize that they still violate closure sometimes. But keep in mind that the support for a perception-relative closure principle that you are claiming to see in both Turri’s data and our data comes from around 3/4 to 4/5 of participants attributing knowledge in accordance with closure in the perception conditions. If this sort of data can be marshaled to support the claim that they endorse closure, why can’t use roughly the same percentages to claim that mathematicians follow closure?
That’s definitely a fair question! Here’s one thought in response. The epistemic closure principle is a necessarily true universal generalization. That is a very strong principle. I think that alternative explanations based on representations of weaker principles would need need to be addressed before reaching for the much stronger one. Does that seem right to you?
I also think that some consideration should be given to the possibility that finding evidence of an epistemic closure principle among highly educated, specialized populations reflects something about that population’s education and specialization, rather than something about folk concepts specifically. To come at this a different way, perhaps the specialized population is applying a different (specialized) concept, rather than doing a better job in applying a folk concept. Again, I’m interested in hearing whether that sounds right to you.
John, the idea that “perhaps the specialized population is applying a different (specialized) concept, rather than doing a better job in applying a folk concept” doesn’t sound right to me. I don’t have much data on the knowledge attributions of mathematicians, but the cases we asked about–your Car Theft and Computer cases–seem to concern ordinary notions. There are cars, parking lots, computer, hackers, and questions about what’s known. Initially at least, it’s not clear to me how a specialized concept might be involved. I’ll have to think about this more.
One way that a specialized concept might enter into things is that mathematicians focus in their professional lives on a particular sort of inference, and it’s not inductive or abductive. This might lead them to think that any inference that’s not a solid deductive proof is second rate, and their opinions in this regard might be more negative than those of the general public–or indeed more negative than experts in other areas where non-deductive inference is the norm (e.g., the sciences). Since the source-relative closure hypothesis concerns inferential beliefs, there might be an issue here.
Very sorry if I misrepresented your position James. I took it that your view was that data from your first two experiments call into question the prior finding closure is sometimes source relative by revealing potential weaknesses of the prior materials used to discover that effect. Was that not correct?
I am a little confused now though. You say you are not trying to question the source relative hypothesis data but explore one explanation of it. The source relative hypothesis says people don’t attribute knowledge in accordance with the unrestricted closure principle. But in your paper you conclude that “an unrestricted closure principle that applies to all beliefs, regardless of source, provides a better model of the patterns of folk knowledge attributions that have been observed”. That makes it sound like you are questioning that.
Wes, I did wonder whether John’s perception condition was a good test of closure, and I had some other concerns–e.g., was not requiring participants to evaluate each knowledge attribution a good idea. However, we note that “inference versions of Car Theft and Computer seem better poised to test for folk endorsement of closure.” And it is with the inference conditions where Turri obtains his closure-violating data. It is this data that we are trying to explain rather than undermine. The source-relative hypothesis says that the reason John obtained the data that he did is that an unrestricted closure principle is not part of folk epistemology. It is this hypothesis rather than the data it is trying to explain that we object to.
Yes I took it that you are attempting to question whether the prior replicable data show what they were claimed to show regarding the hypothesis in questions because either (i) there was something wrong with the materials or (ii) the detected behaviour is due to errors. This is what I meant when I referred to undermining prior findings. Sorry for the confusion.
My comments were intended to question how the present experiments show (i) or (ii) in three ways: first, that the wizard cases are so different from originals it makes inferences about originals difficult; second, that while differences were demonstrated errors were not; third, disputing something being an error means it is not central to practice.